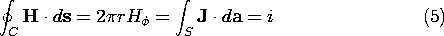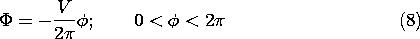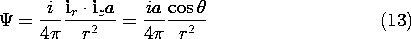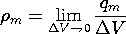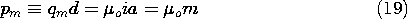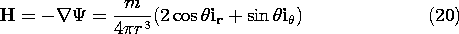8.3
The Scalar Magnetic Potential

The vector potential A describes magnetic fields that possess curl wherever there is a current density J (r). In the space free of current,

and thus H ought to be derivable there from the gradient of a potential.

Because

we further have

The potential obeys Laplace's equation.

Example 8.3.1. The Scalar Potential of a Line Current

A line current is a source singularity (at the origin of a polar coordinate system if it is placed along its z axis). From Ampère's integral law applied to the contour C of Fig. 1.4.4, we have

and thus

It follows that the potential that has H of (6) as the negative of its gradient is

Note that the potential is multiple valued as the origin is encircled more than once. This property reflects the fact that strictly, H is not curl free in all of space. As the origin is encircled, Ampère's integral law identifies J as the source of the curl of H.

Because is a solution to Laplace's equation, it must possess an EQS analog. The electroquasistatic potential

describes the fringing field of a capacitor of semi-infinite extent, extending from x = 0 to x = +, with a voltage V across the plates, in the limit as the spacing between the plates is negligible (Fig. 5.7.2 with V reversed in sign). It can also be interpreted as the field of a semi-infinite dipole layer with the dipole density _s = _s d = _o V defined by (4.5.27), where d is the spacing between the surface charge densities, _s, on the outside surfaces of the semi-infinite plates (Fig. 5.7.2 with the signs of the charges reversed). We now have further opportunity to relate H fields of current-carrying wires to EQS analogs involving dipole layers.

The Scalar Potential of a Current Loop

A current loop carrying a current i has a magnetic field that is curl free everywhere except at the location of the wire. We shall now determine the scalar potential produced by the current loop. The line integral H ds enclosing the current does not give zero, and hence paths that enclose the current in the loop are not allowed, if the potential is to be single valued. Suppose that we mount over the loop a surface S spanning the loop which is not crossed by any path of integration. The actual shape of the surface is arbitrary, but the contour C_l is defined by the wire which is its edge. The potential is then made single valued. The discontinuity of potential across the surface follows from Ampère's law

where the broken circle on the integral sign is to indicate a path as shown in Fig. 8.3.1 that goes from one side of the surface to a point on the opposite side. Thus, the potential of a current loop has the discontinuity

Figure 8.3.1 Surface spanning loop, contour following loop, and contour for H ds.

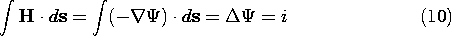
We have found in electroquasistatics that a uniform dipole layer of magnitude _s on a surface S produces a potential that experiences a constant potential jump _s/_o across the surface, (4.5.31). Its potential was (4.5.30)

where 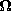is the solid angle subtended by the rim of the surface as seen by an observer at the point r. Thus, we conclude that the scalar potential , a solution to Laplace's equation with a constant jump i across the surface S spanning the wire loop, must have a potential jump _s/_o i, and hence the solution

where again the solid angle is that subtended by the contour along the wire as seen by an observer at the point r as shown by Fig. 8.3.2. In the example of a dipole layer, the surface S specified the physical distribution of the dipole layer. In the present case, S is arbitrary as long as it spans the contour C of the wire. This is consistent with the fact that the solid angle is invariant with respect to changes of the surface S and depends only on the geometry of the rim.

Figure 8.3.2 Solid angle for observer at r due to current loop at r^'.

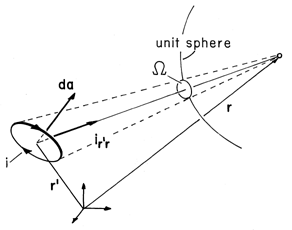
Example 8.3.2. The H Field of Small Loop

Consider a small loop of area a at the origin of a spherical coordinate system with the normal to the surface parallel to the z axis. According to (12), the scalar potential of the loop is then

This is the potential of a dipole. The H field follows from using (2)

As far as its field around and far from the loop is concerned, the current loop can be viewed as if it were a "magnetic" dipole, consisting of two equal and opposite magnetic charges q_m spaced a distance d apart (Fig. 4.4.1 with q q_m). The magnetic charges (monopoles) are sources of divergence of the magnetic flux _oH analogous to electric charges as sources of divergence of the displacement flux density _oE. Thus, if Maxwell's equations are modified to include the action of a magnetic charge density

in units of voltsec/m⁴, then the new magnetic Gauss' law must be

in analogy with

Now, magnetic monopoles have been postulated by Dirac, and recent searches for the existence of such monopoles have been apparently successful

---

² Science Vol. 216, (June 4, 1982).

---

. Because the search is so difficult, it is apparent that, if they exist at all, they are very rare in nature. Here the introduction of magnetic charge is a matter of convenience so that the field produced by a small current loop can be pictured as the field of a magnetic dipole. This can serve as a mnemonic for the reconstruction of the field. Thus, if it is remembered that the potential of the electric dipole is

the potential of a magnetic dipole can be easily recalled as

where

The magnetic dipole moment is defined as the product of the magnetic charge, q_m, and the separation, d, or by _o times the current times the area of the current loop. Another symbol is used commonly for the "dipole moment" of a current loop, m ia, the product of the current times the area of the loop without the factor _o. The reader must gather from the context whether the words dipole moment refer to p_m or m = p_m/_o. The magnetic field intensity H of a magnetic dipole at the origin, (14), is

Of course, the details of the field produced by the current loop and the magnetic charge-dipole differ in the near field. One has _o H 0, and the other has a solenoidal H field.