Back to online papers
Colors and Reflectances
Alex Byrne and David R. Hilbert
[Published in Readings on Color, Volume 1: the philosophy of color, MIT Press, 1997]
1 Introduction
When we open our eyes, the world seems full of colored opaque objects, light sources, and transparent volumes. One historically popular view, eliminativism, is that the world is not in this respect as it appears to be: nothing has any color. Color realism, the denial of eliminativism, comes in three mutually exclusive varieties, which may be taken to exhaust the space of plausible realist theories. Acccording to dispositionalism, colors are psychological dispositions: dispositions to produce certain kinds of visual experiences. According to both primitivism and physicalism, colors are not psychological dispositions; they differ in that primitivism says that no reductive analysis of the colors is possible, whereas physicalism says that they are physical properties. This paper is a defense of physicalism about color.
We shall proceed as follows. After first making a useful distinction and some preliminary clarifications immediately below, we outline and motivate our own theory in section 2. Then, in section 3, we reply to three objections. The case for physicalism is summarized in section 4.
1.1 Green-Representing and Green-Feeling Experiences
If it looks to you as if something is green and square at location L, then your experience is veridical only if something is green and square at L. If in fact there is nothing green and square at L (perhaps there is only a green circle or a pink square at L, or perhaps there is only pink circle, or even nothing at all) then your experience is (at least partly) illusory. Thus your experience is veridical only if the proposition that there is something green and square at L is true, and illusory otherwise. It may also look to you as if something is orange and round at location L´. Thus your experience is veridical only if the proposition that there is something orange and round at L´ is true, and illusory otherwise.
Your experience, then, may be said to represent that there is something green and square at L, and that there is something orange and round at L´. If we take propositions to be sentence-like entities, we may define a conjunctive proposition C -- the content of your experience -- as follows. A proposition p is a conjunct of C iff p is represented by your experience (taking C to be a conjunct of itself, and "represents" to distribute over conjunction). Intuitively, the content of a visual experience is the complete way the experience represents the world as being.
Typically, you will accept "the testimony of your senses", and believe that there is something green and square at L. If you come to believe that the lighting conditions are abnormal (for example), you may change your mind, and not believe that the world is this way. But even so, it will continue to look as if there is something green and square at L -- the proposition that there is something green and square at L will continue to be represented by your experience. There is no straightforward relationship, then, between the content of an experience and the content of beliefs formed on the basis of that experience.
Let a green-representing experience be a visual experience that represents the world as containing something green. Thus the content of such an experience will have as a conjunct a proposition that predicates the property green.[1]
Now, when people with normal vision look at grass, shamrocks, and jade, in daylight, they have green-representing experiences. These experiences are thus similar in respect of content. Assuming, as we shall, that "spectrum inversion" does not actually occur, such experiences are also phenomenologically alike: there is something obviously similar in respect of what it is like to undergo them. Let a green-feeling experience be a visual experience with this phenomenological character.
Whether green is a physical property or even whether anything is green, at least this much is true: typically, whenever someone has a green-feeling experience, she has a green-representing experience.[2] That is, the property green is a property that green-feeling experiences typically represent objects as having.[3] However, what is very much in dispute is whether this connection between green-feeling experiences and green-representing experiences is necessary. Many think, for example, that in some possible worlds green-feeling experiences represent, not that objects are green, but that they are red. We shall be taking up this question later.
So, let it be clear at the outset that our main interest is in properties that certain types of (human) visual experiences represent objects as having. We are not significantly concerned with the question of whether other animals see colors and, if so, what sort of properties these might be (on this latter topic, see Hilbert 1992). Neither are we significantly concerned with the semantics of color words. And we are not at all concerned with an "objective physical definition" (MacAdam 1985, p. 34) of color that can be employed in good conscience by color scientists irrespective of how matters stand with visual experience.
2 A Physicalist Theory of Color
Our physicalist theory of color has two main components. First, colors are types of surface spectral reflectances. Second, color content and color phenomenology necessarily go together. We shall discuss these in turn.
2.1 Colors Are Reflectances
The surface spectral reflectance (SSR) of an object is given by specifying, at each wavelength in the visible spectrum, the percentage of light the object reflects at that wavelength. As one of us has argued at length before, the most plausible version of physicalism about surface (or object) colors takes these properties to be types of SSRs.[4] For simplicity we will concentrate here on surface color[5]; the extension of our account to light sources and transparent volumes is a matter for another time.[6]
If two objects have the same SSR, in all visible illuminations they will reflect the same amount of light. If one object is substituted for another with the same SSR (assuming they are the same size) in the scene before the eyes, no visible color difference will result. The SSR of an object is (typically) an illumination-independent property: the SSR of an object does not change if the object is taken from a room to a sunny street, or if the lights are turned out. And this, arguably, is also a feature of color.
Let us assume that our visual experiences are, in normal viewing conditions, mostly veridical in respect of color. Certain frogs, iceberg lettuce, and dollar bills (say) are green. Is there an SSR that all and only green objects share? Given that our color perceptions are mostly veridical, the answer is no. Green objects differ widely in their SSRs. This is even true for particular shades of green, as is shown by the phenomenon of metamerism: the fact that some objects with different SSRs have perceptually indistinguishable colors in normal conditions of illumination. Two objects that differ in reflectance but have perceptually identical colors in a certain illumination are a metameric pair (with respect to that illumination).[7] Moreover, the difference in reflectance between the members of a metameric pair need not be small in the way that the difference in length between two lines of perceptually indistinguishable length is small. An object that reflects almost no light in the neighborhood of 540 nanometres (nm) could be indiscriminable in normal conditions of illumination from an object that reflects nearly all the incident light at this wavelength.
But is there a physical property that all and only (actual and possible) green objects share? Given our assumption about the general correctness of our color perceptions, the answer is (plausibly) yes. The property that all green objects share is a type of SSR.[8] Very roughly, this property -- call it "SSRGREEN" -- is the type of SSR that allows an object in normal illumination to reflect significantly more light in the middle-wavelength part of the spectrum than in the long-wavelength part, and approximately the same amount of light in the short-wavelength part as in the rest.[9] Obviously, particular reflectances meeting these specifications -- for instance those of frogs, lettuce, and dollar bills[10] -- may be otherwise very different.
The property green, if it is this type of SSR, is not a particularly interesting property from a physical point of view. Since we only find it salient because our perceptual apparatus is built to detect it, it might be called an anthropocentric property (cf. Hilbert 1987). Alien physicists lacking our visual apparatus would not need to single it out for special attention, unlike the property of having charge e, or spin 1/2. (These aliens might likewise find the visible spectrum no more than an arbitrary segment of the entire electromagnetic spectrum.) But that does not at all impugn the status of an idiosyncratic type of SSR as a physical property that is "objective," in almost every sense of that protean word. Particular SSRs are not in any philosophically interesting sense dependent on human beings; neither is the type, "either SSRa or SSRb or SSRg, ... ," where these particular SSRs seem from a physical standpoint to be a motley collection.
As for the hues, so for the other color categories. The determinable green has various determinate shades -- olive green, lime green, and so on. And it is itself a determinate of the determinables chromatic color, and color. These are all types of reflectances.
Let SSRGREEN be a set of SSRs, such that an SSR is a member of this set just in case it is of the type we sketched a few paragraph back -- SSRGREEN.[11] And in general: for any color X (a type of SSR), a particular SSR will be of this type just in case the SSR is a member of SSRX. If we like, we can call the individual SSRs the "maximally specific colors," although it must be stressed that this is simply a natural way of extending our everyday color talk. The reflectance-types that the human visual system represents objects as having are considerably coarser than the maximally specific colors. Hence, although of course objects having maximally specific colors are visible, the maximally specific colors themselves are not, because they are not properties that one can tell an object possesses simply by looking at it. That is why the terminology is an extension of ordinary usage.[12]
This set-theoretic apparatus gives us a nice way to explain the determinate-determinable relation. But first we need a distinction. A color property is simply a reflectance-type. Thus, assuming our version of physicalism, examples of color properties are red, bluish green, sky blue, purple-or-green, sky blue-or-bluish-green-or-pink, and the maximally specific colors.
Now some color properties are those that objects can look to have, and others are not. An object can look lemon yellow, yellow, chromatically colored, or simply colored, but to humans nothing can look purple-or-green, or to have a maximally specific color. (Admittedly, one can tell simply by looking that an object is purple-or-green, but that is not to say that the object looks purple-or-green; rather, it will look green, or look purple, and thus one may infer that the object is purple-or-green.)
Those color properties that objects can look to humans to have will be precisely those that human visual experience can represent objects as having. Say that such properties are representable color properties.
With this distinction between color properties (like green) that are representable and those (like purple-or-green) that are not, we can say that two color properties, X and Y, stand to one another in the relation of determinate to determinable just in case X and Y are representable color properties and SSRX is a proper subset of SSRY. So lime green is a determinate of green, because this property (and green itself) is a representable color property, and SSRLIME-GREEN is a subset of SSRGREEN. But, although SSRPURPLE is a proper subset of SSRPURPLE-OR-GREEN, purple-or-green is not a representable color property and hence, as desired, not a determinable of purple.
2.2 Content and Phenomenology
The relationship between the content and phenomenology of experience has recently been much debated.[13] Any adequate theory of color must take a stand on this question, at least as it concerns color experience.
Let us say that two experiences are the same in color content just in case they represent the same color properties instantiated at the same (viewer-centered) locations. And let us say that two experiences are the same in color phenomenology just in case (to put it loosely and intuitively) any phenomenological difference between them would not be described using color vocabulary. (Thus, the phenomenological difference between seeming to see movement at the periphery of one's field of view and not seeming to see such movement is not a difference in color phenomenology.)
We may now put the thesis we wish to defend as follows:
NECESSITY
For all possible subjects S1, S2 and all possible worlds w1, w2, if S1 is having a visual experience in w1 and S2 is having a visual experience in w2, then these experiences are the same in color content iff they are the same in color phenomenology.
That is, we claim that the color content and color phenomenology of visual experience cannot come apart. (It is worth noting that Boghossian and Velleman's attack on physicalism in chapter 8 of this volume is largely directed against versions that hold color content and color phenomenology to be only contingently connected.[14])
NECESSITY is intuitively plausible, at least in straightforward cases of vision. The experience of seeing a ripe tomato does not seem to contain content and phenomenology as separable elements. Asked, first, to imagine having a visual experience that represents the world as containing a red tomato immediately before one and, second, to imagine having a visual experience whose color phenomenology is like that, the naive subject has only one way of responding to both questions.
Why think NECESSITY is false? There are a number of apparent counterexamples. Of these, only one -- the case of "spectrum inversion" -- requires extended discussion. The rest we shall relegate to a footnote.[15]
Imagine, then, that Invert and Nonvert are "spectrally inverted" with respect to each other, and have been since birth. Nonvert has normal vision, but Invert has red-feeling experiences when he looks at gooseberries, and green-feeling experiences when he looks at raspberries. First premise: this case is possible. Second (relatively uncontroversial) premise: Nonvert's experiences represent, by and large, the true colors of objects. Third premise: the same is true of Invert (cf. Shoemaker 1982; Block 1990). If these three premises are true (and we shall examine how the first and third might be supported shortly), then we have counterexamples to both the left-to-right and right-to-left parts of NECESSITY, as follows.
The left-to-right part. When Invert and Nonvert both look at a gooseberry, their experiences are both green-representing. Thus this is a case of same color content (the gooseberry is represented as having the property green), but different phenomenology (Invert and Nonvert have, respectively, red- and green-feeling experiences).
The right-to-left part. When Invert looks at a gooseberry and Nonvert looks at a raspberry, they both have red-feeling experiences that are, respectively, green- and red-representing: same phenomenology, different color content.[16]
Our response is this. First, we shall describe a hypothetical case of a subject -- Fred -- who is spectrally inverted in only one eye, using only assumptions that the inverted spectrum argument itself requires. Then we shall imagine that Fred is presented with a red raspberry, and looks at it first through one eye, and then through the other. His visual experience will change. If the inverted spectrum argument against NECESSITY is sound, then (it turns out) this change in Fred's experience is not a change in the color properties the raspberry is represented as having. We shall argue that this is not acceptable. Hence the inverted spectrum argument is unsound.[17] We shall finally discuss the question of which premise of the inverted spectrum argument should be denied.
To begin. Suppose that Fred is spectrally inverted in only one eye, his left. So, he has red-feeling experiences when he looks at green objects with his left eye, and green-feeling experiences when he looks at green objects with his right eye. Further imagine that Fred only uses one eye at a time (say the left on Monday, Wednesdays, and Fridays, and the right the other days of the week). He has been raised in an environment that changes color early on Monday, Wednesday, and Friday mornings, and changes back on Tuesday, Thursday, and Saturday. These changes amount to successive color inversions and reinversions: before dawn on Monday, gooseberries change from green to red, and raspberries change from red to green; before dawn on Tuesday gooseberries change back to green, and raspberries change back to red.
Gooseberries, then, produce green-feeling experiences in Fred any day of the week; likewise, mutatis mutandis, for other objects. So we may fairly suppose that Fred believes he is cyclopean, and has no inkling that his environment changes color in this systematic way.[18] It seems reasonable to presume that, if Invert's phenomenological inversion with respect to Nonvert is possible, Fred's case, as we have described it so far, is likewise possible.
Now, on the assumption that Invert's visual experience, when he is looking at a gooseberry, represents the gooseberry as having the property green, and so forth, what are we to say about the content of Fred's visual experiences?
Well, why is it supposed that Invert sees the true colors of objects? Here we should distinguish two reasons. First, it might be claimed that this is simply an intuitive judgment about the case -- after all, Invert can use his color vision to navigate the world successfully, so why suppose he is systematically misperceiving it? If this is the reason, then it seems we may draw the same conclusion in Fred's case (perhaps, because of the additional complexity, a little more tentatively): so, for example, when Fred looks at a (green) raspberry on Monday with his left eye, his red-feeling experience is green-representing.
Second, it might be argued that Invert sees the true colors of objects from the theoretically motivated premise that color content is an extrinsic matter: it varies between subjects who are duplicates, and so can be affected by purely environmental changes.[19] Putnam's familiar Twin Earth story shows that this is so in the case of contents involving natural kind concepts, like the concept of water (Putnam 1975). On Earth Oscar believes that water is wet. On Twin Earth, where the clear potable fluid XYZ flows in the rivers and falls from the sky, and where H2O is nowhere to be found, his perfect twin Twoscar does not have this belief. He believes that twater (the stuff XYZ) is wet.
For simplicity, let us suppose that the diagnosis of this difference in content is that an inner state-type of Oscar is reliably caused by the presence of water, not twater, and vice versa for Twoscar. If the moral of Twin Earth extends to Invert's case, then because his red-feeling experiences are reliably caused by the presence of green objects before his eyes, he has a green-representing experience when he looks at a gooseberry.
If all this is right, then it would seem that red-feeling experiences produced by Fred's left eye are green-representing. For they are reliably caused by the presence of green objects -- Monday's raspberries, for instance. And we may suppose that the visual pathways leading from each of Fred's eyes do not causally interact. He thus has, in effect, two visual systems used on different days, and that is presumably enough to apply the simple externalist theory of content mentioned above to each individually.
(Of course, externalist theories of content may take other forms (see, for example, Millikan 1984; Dretske 1981, 1988; Fodor 1990). But whatever the details, it seems very likely that we can have our desired result -- that green-feeling experiences produced by Fred's left eye, and red-feeling experiences produced by his right, are red-representing -- at the cost of complicating our example.)
The upshot, then, is that if Invert is not the victim of a systematic color illusion, by parity of reasoning Fred isn't either.[20]
So, if the standard inverted spectrum case is a genuine counterexample to NECESSITY, Fred's plight may be described as follows. He has his left eye phenomenologically inverted with respect to his right: red objects viewed with his left eye cause green-feeling experiences, and red-feeling experiences when viewed with his right. But Fred's green-feeling experiences produced by his left eye are red-representing, just like the red-feeling experiences produced by his right eye.
Now for the advertised change in Fred's experience that we say a proponent of the inverted spectrum argument must misdescribe. Suppose that we take a red raspberry and allow Fred to look at it first with his right eye, and then with his left (contrary to his usual practice). What will Fred's visual experience be like? Well, simply imagine a raspberry that changes color from red to green, and imagine looking at the raspberry through one eye, blinking just as the raspberry changes color. Assuming you have normal vision, that is what it will be like for Fred.
To Fred it will be as if the raspberry -- part of the scene before his eyes -- has undergone a change. If Fred thinks conditions are normal, and that his visual apparatus is functioning properly, he will believe, on the basis of his experience, that the raspberry has changed in respect of a salient surface property. That is, the raspberry first looks one way to Fred, and then another way.
But of course this is precisely what a defender of the inverted spectrum argument cannot say. The difference between Fred's first and second look is not a difference in the properties his experience represents objects as having. If the inverted spectrum argument is sound, the "testimony" of Fred's experience is that the world is exactly the same way both times, that the raspberry has not changed at all. Surely this cannot be correct. The fact is that the scene before Fred's eyes looks to him to change (it is irrelevant that the appearances are deceptive). But this just is a change in the content of his experience, at least if we are to retain any intuitive grip on that notion.[21],[22]
The inverted spectrum argument is therefore unsound. According to the first premise, the phenomenology of Invert's color experiences is inverted with respect to Nonvert's. According to the second premise, Nonvert sees the true colors of objects. According to the third premise, Invert's visual experiences are likewise veridical. As we have indicated, in the present context the second premise may be taken for granted. We now need to examine what either denying premise one, or denying premise three, would involve.
As we mentioned, two reasons might be given for holding the third premise: that Invert's experiences represent raspberries as having the property red, gooseberries as having the property green, and so on. First, that this is more or less obvious just from the description of the case. Second, that color content is an extrinsic matter.
We may similarly distinguish two reasons for holding the first premise: that Invert's alleged long term phenomenological inversion is possible. First, that this is more or less obvious just from the description of the case. Second, that phenomenology is an intrinsic matter: it does not vary between subjects who are duplicates, and so is unaffected by purely environmental changes. According to this second reason, there is a certain intrinsic state that is sufficient for having a green-feeling experience, and Invert is hooked up to the world in such a way that a raspberry before his eyes causes him to be in it.
So there are two reasons -- intuitive and theoretical -- for each of the first and third premises. And therefore we need to deny both the intuitive and theoretical reasons for one of them. If it's the third premise, then we must say that (a) any intuition that Invert sees the true colors of objects is not probative, and (b) the content of color experience is intrinsic[23] -- it does not vary between duplicate subjects. If it's the first premise, then we must say that (c) any intuition that Invert's phenomenological inversion is possible is not probative, and (d) the phenomenology of color experience is extrinsic -- it does vary between (some) duplicate subjects.
And here, it might be thought, we face a dilemma, because (a), (b), (c), and (d) are widely taken to be very implausible. But each of thinks he can comfortably sit on one of these horns.[24] Although we concede that both premises have some measure of intuitive support, it is surely far from conclusive, and thus a case can be made for (a) and (c). (Admittedly, it would be nice to have an explanation of why intuition has led us astray.) What about (b) and (d)? First, whatever may be the case with water-beliefs, it is quite disputable that color content is extrinsic. Not all theories of intentionality worth taking seriously exclude intrinsic content across the board.[25] To take a crude example, consider the theory that the mental symbol "red" refers to the property red because in ideal conditions instantiations of red would cause tokenings of the symbol "red," and similarly for the other colors. Provided that the specification of "ideal conditions" is wholly determined by the intrinsic properties of the subject, as it conceivably might be, twins will share color contents, and so color content will be intrinsic. We do not think that anyone is in a position to rule out all possible versions of such theories. Second, it is also quite disputable that phenomenology is intrinsic (for recent dissent, see Dretske 1995, 1996; Tye 1995; Lycan 1996).
3 Replies to Objections
So far we have set out, with some accompanying defence, the main lines of our own theory. In this section we reply to three objections. The first is (in effect) an objection to NECESSITY. The second two are common objections that are widely taken to be fatal to any sort of physicalism about color.
3.1 First Objection: Actual Variations in Phenomenology
When subjects with normal vision are asked to locate "unique" green on the spectrum -- a green that is neither yellowish nor bluish -- the variation in their responses is significant, at least covering the interval from 490 to 520 nm. And disputes about whether, say, a bluish green fabric is predominantly green or predominantly blue are common in everyday life.
Take two normal subjects, Ted and Alice, who disagree on the spectral location of unique green. Ted says it's 490 nm, Alice says it's 520 nm. It is natural to suppose that Ted and Alice have phenomenologically identical experiences when looking at, respectively, 490 and 520 nm lights. And it is equally natural to suppose that they have phenomenologically different experiences when they look at 490 nm lights. To avoid complications with the colors of light sources, let's switch attention to object color. There will be a patch that produces in Ted a unique-green-feeling experience, but produces in Alice a bluish-green-feeling experience.
By NECESSITY, since our subjects enjoy phenomenologically different experiences, the color contents of their experiences must differ. Ted's experience is representing the patch to be unique green, and Alice's experience is representing the patch to be bluish green. These are different properties, and (so the argument goes) they are contraries: if something is unique green, it is not also bluish green. So either Ted or Alice is misperceiving the color of the patch. This, it might be thought, is implausible.
According to us, unique green is a type of SSR -- SSRU-GREEN. And an SSR is of this type just in case it is a member of SSRU-GREEN. Likewise, bluish green -- let's pick a particular shade -- is a reflectance-type whose corresponding set is SSRB-GREEN. So far, none of this helps. But suppose that SSRU-GREEN and SSRB-GREEN intersect -- they have some members in common. Then although Ted and Alice's visual experiences represent the patch to have different properties, those properties are not contraries. Therefore, if this supposition is reasonable, there is no barrier to supposing that both Ted's and Alice's experiences are veridical. We cannot prove, of course, that this supposition is correct, but we do claim that it is a possibility to be taken seriously.
But is it reasonable? Surely nothing can be both unique green and bluish green! But why not? Of course, we are not denying that unique green and bluish green are distinct properties. Nor are we denying that nothing can appear simultaneously to one perceiver to be both unique green and bluish green. We are suggesting, though, that some ways of being unique green are also ways of being bluish green.
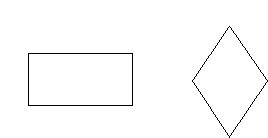
One way to show that this is an option is to find other perceptible properties that are partners in crime. Take the experiences of, on the one hand, something's looking rectangular and, on the other, something's looking diamond-shaped. When one enjoys the former type of experience, the symmetries about the bisectors of the figure's sides are salient. And when one enjoys the latter type of experience, the symmetries about the bisectors of the figure's angles are salient.[26] For an example of the types of experiences we mean, look at figure 14.1.

Figure 14.1
Now, although the property of being a diamond and the property of being a rectangle are not contraries, arguably nothing can look simultaneously to be a rectangle and a diamond. Squares, of course, are both diamonds and rectangles. But no square both looks to be a rectangle and a diamond simultanously. Not, at any rate, in the particularly obvious way illustrated by figure 14.2.
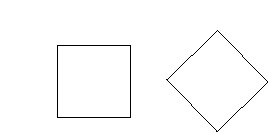
Figure 14.2
Here the left-hand figure looks rectangular, and the right-hand figure looks diamond-shaped. It is not perceptually obvious that the left hand figure is diamond-shaped, or that the right-hand figure is rectangular. It would not be altogether surprising to find a perceiver on a restricted diet of shape perceptions who mistakenly thought that no diamond could ever be rectangular.
But isn't there this disanalogy with the color case? By physically rotating figure 14.2, or by rotating the corresponding mental image, one can readily see that the right-hand figure is rectangular. That is why the idea that the properties of being a square and of being a diamond are contraries does not survive informed reflection. But if a patch looks unique green, that's the end of the matter.
Not quite. If a patch looks unique green, it can typically be made to look bluish green by changing the viewing conditions slightly. Admittedly, there is nothing that is exactly parallel to the rotation of a mental image, but the analogy does not have to be perfect. The point is simply to show that two shape properties could mistakenly seem to be contraries, and hence that the corresponding claim in the color case is at least a possibility.
But even if bluish green and unique green are in fact contraries, this is not a disaster. That many of us misperceive unique green objects is certainly an unwelcome result; but at least (for all the objection says) we veridically perceive them as green, and perhaps that is enough.
3.2 Second Objection: Red Is Really More Similar to Orange Than It Is to Green
Before stating this objection, we need to introduce two senses of similarity.
First, relative similarity. Let x, y, and z be distinct things (x is not equal to y is not equal to z). x and y are perfectly similar (or just plain similar) relative to a family of properties {P1,..., Pn} just in case both x and y have each Pi. x is more similar to y than to z, relative to a family of properties {P1,..., Pn} just in case x and y share more Pis than x and z do.[27]
So, for example, a square and a triangle are both similar relative to the family {having more than three angles}, and are also both similar relative to the family {being either a square or a triangle}. (This last relative similarity claim shows that any two things are similar relative to some property or other.[28]) Properties themselves have properties, and so, like particulars, stand in various relative similarity relations. For example, the property squareness and the property triangularity are similar relative to the family {being a geometrical property}.
Here are two examples of comparative relative similarity. A square is more similar to a circle than to a triangle, relative to the family {having at least fourfold symmetry, being a nontriangle, having exactly three angles}. A square is more similar to a triangle than to a circle, relative to the family {being angled, being a closed figure, having no straight edges}.
One might think that relative similarity is all the similarity there is, but this is arguably not the case. Think of perfect duplicates -- this cube of sugar and a molecule-for-molecule copy of it. They share all their intrinsic properties. Isn't this a natural respect in which they are similar? Suppose we have cube, a tetrahedron, and a sphere. Isn't the first more similar, in a natural respect, to the second than it is to the third? Again, isn't squareness more similar, in a natural respect, to triangularity than it is to circularity? Assuming these questions are at least intelligible, let us call similarity in a natural respect, natural similarity.[29]
Let SIMILARITY be the claim that -- in the natural sense -- red is more similar to orange than to green. Now we can set out the objection in the form of an argument[30]:
(1) We know SIMILARITY solely on the basis of ordinary visual experience.
If the colors are physical properties -- the argument continues -- then we would need to find out which physical properties red, orange, and green are, in order to determine whether or not SIMILARITY is true. That is, we would need color science -- ordinary visual experience wouldn't suffice. So:
(2) If physicalism is true, (1) is false.
Hence:
(3) Physicalism is false.
It must be admitted that the first premise appears to be in good shape. The first premise (and so SIMILARITY) seems true, perhaps even obviously so. Indeed, theses like SIMILARITY are often given as paradigmatic examples of natural similarity relations holding among properties.[31]
What about the second premise? We may well regard the above reasoning in its support with some suspicion. Admittedly, if colors are physical properties, then SIMILARITY is hostage to empirical fortune. Color science, we may fairly presume, reveals the nature of properties like reflectances, and thus reveals the natural similarity relations in which reflectances stand to each other. And there can surely be no guarantee that these similarity relations will be the ones we believe to hold on the basis of ordinary perception. However, the mere possibility of defeat does not generally overturn claims to knowledge -- why should it do so in this case? To this there are two replies. First, it might be argued that there is no reason to suppose that ordinary perception is a reliable guide to natural similarity relations between physical properties. If so, physicalism implies, not just the possibility of defeat, but that we have no business believing SIMILARITY simply because red, orange and green look that way. According to this first reply, if physicalism is true, then we are not justified in taking SIMILARITY to be true solely on the basis of ordinary perception, and thus we do not know it by these means. Second, it might be argued that our claim to know SIMILARITY has a special status: it is simply not defeasible. According to this second reply, the first premise should be reformulated along these stronger lines. Then, since physicalism implies that our claim to know SIMILARITY is defeasible, the argument for the second premise is straightforward.
We need not pursue this any further, because if physicalism (our brand of it, at least) is true, some intuitively correct natural similarity claims in the style of SIMILARITY will be false. Perhaps SSRRED is more similar, in the natural sense, to SSRORANGE than to SSRGREEN; but it seems extremely doubtful that this relation holds between, for example, SSRBLUE, SSRPURPLE, and SSRGREEN.[32] Hence the truth of (a variant of) the second premise is immediate. Physicalism implies that we couldn't know that blue is more similar to purple than to green without color science, for the simple reason that blue is not more similar to purple than to green, if physicalism is true. So it appears that we are in big trouble.
We deny the first premise. Visual experience, we think, does not tell us that the colors are naturally similar. Rather, visual experience tells us that colored objects in the scene before the eyes are relatively similar -- relative to the color properties that one's experience represents the objects as having. This needs some explaining.
A certain colored chip looks to be a maximally determinate shade of red -- call it "red21." It also looks scarlet (say), red, chromatically colored, and colored. So the visual experience of the chip not only represents it as having red21, but also as having, inter alia, the color properties scarlet, red, chromatic color, and color. Thus, assuming physicalism, the chip is visually represented as having various types of reflectances, ranging from the comparatively determinate SSRRED21 to the maximally indeterminate SSRCOLORED, where any object that falls under the more determinate reflectance-types also falls under the less determinate ones. (Recall the end of section 2.2. above.)
Now suppose that you see three chips -- x, y, and z -- together, and that x looks scarlet, y crimson, and z lemon yellow. You would judge that x is more similar (in respect of color) to y than to z. Why? An explanation is to hand if we make a natural assumption about the sorts of color properties that your experience represents the chips as having. Namely, there are more color properties that x and y are both represented as having than there are color properties that x and z are both represented as having. If that were so, then x would be seen as sharing more color properties with y than with z. And this would make your comparative similarity judgment intelligible.
That assumption seems right in this case. Indulging in some oversimplification, the color properties that x and y are both represented as having are: red, chromatic color, and color. The color properties that x and z are both represented as having are: chromatic color, and color. And there are more of the former than the latter.
So we propose that our judgments of color comparative similarity relations between objects are simply reflections of relative similarity relations holding between those objects. Let C1, C2,... be all the color properties. Suppose x is judged more similar (in respect of color) to y than to z, on the basis of experience E. Then this judgment is explained by the fact that x is more similar to y than to z, relative to the family {being visually represented by E as having C1, being visually represented by E as having C2,... }. But what is the content of the judgment that x is more similar (in respect of color) to y than to z? Let an E-color property be a color property that E actually represents either x or y or z as having. Then we may analyse -- or, perhaps better, explicate -- the judgment that x is more similar (in respect of color) to y than to z thus: x shares an E-color property with y but not z, and every E-color property x shares with z it shares with y.
It is a good deal less clear what people are up to when they make similarity claims about color properties, for instance, that scarlet is more similar to crimson than to lemon yellow. Some of them, at least, will mean to be making a natural similarity claim. (In which case, according to us, sometimes they will speak falsely.) We have already explained why, when confronted with any three objects, looking scarlet, crimson, and yellow, respectively, a subject will judge the first more similar to the second than the third. Any reflective subject knows that any three such objects will elicit such a similarity judgment. And, without getting into the question of whether subjects speak truly when they say that scarlet is more similar to crimson than to lemon yellow, this is enough to explain why they say it.
To make this explanation of our similarity judgments between color properties quite general, it will be useful to define a relation between three color properties X, Y, and Z as follows:
X looks more similar to Y than to Z iff for all possible visual experiences E[33], and for all possible objects x, y, and z, if E represents x as having X, y as having Y, z as having Z, then:
There are more color properties that E represents x and y as having than there are color properties that E represents x and z as having.
Then we can say that our explanation of judgments of similarity between the colors is this: any intuitively true similarity claim of the form "X is more similar to Y than to Z" (setting aside whether it is true) corresponds to a truth of the form "X looks more similar to Y than to Z", and vice versa.
Now someone might object that this is not always so. For consider red, orange, and green. Suppose x, y and z are seen as having these colors, respectively. x is represented to be various more or less determinate shades of red, red, chromatically colored, and colored. y is represented to be shades of orange, orange, chromatically colored, and colored. z is represented to be various shades of green, green, chromatically colored, and colored. If this is the full story, then the color properties x and y are both represented as having are the color properties that x and z are both represented as having, viz. chromatic color and color. Thus, if we look back at how "red looks more similar to orange than it does to green" is defined, we see that it's false. But of course "red is more similar to orange than it is to green" is an intuitively true similarity claim. This looks like a difficulty for our account.
But it can be overcome. It is reasonable to suppose that there is a color property that x and y, but not z, are represented as having, and if so the above account of the color properties x, y, and z are represented as having is incomplete. The common property is red-or-orange-or-purple -- "reddishness," for short (we are taking pink to be a kind of red and brown a kind of orange). Anything that's either red or orange or purple does look (as we say) reddish. Therefore the natural supposition is that there is a property that visual experience represents all red, orange, and purple objects (and no others) as having.
According to the proponent of the argument we have just been considering, visual experience tells us that red is more similar to orange than to green, in the natural sense. We say it tells us nothing of the sort. Visual experience simply tells us that red objects have certain color properties in common with orange objects, and certain color properties in common with green objects; and there are more of the former than the latter.
It must be emphasised that we are not explaining why or how the visual system represents objects as having this multitude of properties. That might be thought a disadvantage, but in any case we are not denying anything that is known at the neurophysiological level, or taking a stand on the details of opponent-process theory.
One advantage of our account is that it makes no appeal to the notion of natural similarity relations among properties. It is not so clear that the idea is intelligible, and in any case has proved very hard to analyze[34]. A fortiori, we do not need to suppose that visual experience can represent such relations.
3.3 Third Objection: Physicalism Cannot Account for Binary Structure
Hardin has claimed that even if physicalism is successful in accounting for the relations of similarity and difference among the colors, there still remains one crucial sort of chromatic fact that resists a physicalist treatment. As we have already noted, some colors, for example, orange, always look to be mixtures, whereas others, for example, red and yellow, do not. Any shade of orange appears, or at least will appear to a reflective perceiver, to be a mixture of red and yellow in varying proportions. In the case of yellow, however, there is a shade of yellow that does not appear to be a mixture of any other hues -- unique yellow. There are exactly four unique hues (red, green, yellow, blue) and all the others are binary.[35] This distinction is deeply embedded in contemporary color science and is thought by many to reflect fundamental facts about the physiology of color vision.[36] Here, Hardin argues, we have a serious problem for physicalism:
If we reflect on what it is to be red, we readily see that it is possible for there to be a red that is unique, i.e., neither yellowish nor bluish. It is equally apparent that it is impossible for there to be a unique orange, one that is neither reddish nor yellowish...If yellow is identical with G, and orange is identical with H, it must be possible for there to be a unique G but impossible for there to be a unique H. If hues are physical complexes, those physical complexes must admit of a division into unique and binary complexes. No matter how gerrymandered the physical complex that is to be identical with the hues, it must have this fourfold structure, and, if objectivism [i.e. physicalism] is to be sustained, once the complex is identified, it must be possible to characterize that structure on the basis of physical predicates alone (1993, p. 66).[37]
Let BINARY be the claim that yellow is unique and orange is binary. The passage from Hardin suggests an argument paralleling the one in the previous section:
(1) We know BINARY solely on the basis of ordinary visual experience.
If -- the argument continues -- yellow and orange are "physical complexes," and if BINARY is true, "those physical complexes must admit of a division into unique and binary complexes." Hence, if the colors are physical properties, then we would need to find out which physical properties are yellow and orange, in order to determine whether or not BINARY is true. That is, we would need color science -- ordinary visual experience wouldn't suffice. So:
(2) If physicalism is true, (1) is false.
Hence:
(3) Physicalism is false.
Unlike the previous argument, where SIMILARITY had a tolerably clear interpretation, the usual explanation of BINARY is more than a little opaque. Take orange. We say it is a binary color because it is, or appears to be, a mixture of red and yellow. But what does that mean? Is orange a combination of the two properties red and yellow? No: a "combination" of two properties A and B is presumably the property A&B (if it's not that, what is it?). Everything that has the property red&yellow is red, but (many) orange objects are not red. Is orange a mixture of red and yellow as a field of poppies and buttercups is a mixture of red and yellow? No, because an orange patch does not appear composed of separate red and yellow blobs. Is orange binary because any orange pigment looks as if it was formed by mixing red and yellow pigment? Well, given common experience with pigments, and a mildly theory laden account of perception, perhaps it does appear that way. But this can hardly be in what the binary/unique distinction consists.[38] If there is a sense in which orange pigment looks to be a mixture of red and yellow pigment, then presumably green pigment looks in this sense to be a mixture of yellow and blue pigment. If this explanation of the binary/unique distinction were right, green would be a binary color. But it isn't.
So it is really not at all obvious that there is a natural interpretation of BINARY such that its truth requires some physically motivated "division into unique and binary complexes."[39] In any case, we have an analysis of BINARY that shows that, even if physicalism is true, it may be known on the basis of ordinary visual experience. Thus we deny (2).
In the previous section we claimed that there is a color property -- reddishness -- that visual experience represents all and only red, orange, and purple objects as having. Therein lies the similarity between these colors. There is equal reason to believe that visual experience represents objects as having greenishness, blueishness, and yellowishness.
Setting aside color properties that are always represented in (chromatic) color experience -- chromatic color and color -- the four properties just mentioned are plausibly the superdeterminables: every other representable color property is a determinate of one of these properties.
Now any object that is visually represented as orange is also represented as having precisely two of these superdeterminables, reddishness and yellowishness (in fact, we may identify orange with reddishness&yellowishness). And any object that is visually represented as yellow is either represented as having greenishness and yellowishness, reddishness and yellowishness, or, in the case of unique yellow, only yellowishness. Thus, there is a shade of yellow such that any object represented as having that shade is represented as having just one superdeterminable, and no such shade of orange. This is our analysis of BINARY. And so there is no evident difficulty in knowing that yellow is unique and that orange is binary on the basis of ordinary visual experience. The objection from binary structure therefore fails.[40]
4 Conclusion: The Case for Physicalism
Physicalism about color has considerable attractions. Unlike eliminativism, it does not convict experience of widespread error. Unlike primitivism, it provides a reduction of the colors and, moreover, a reduction to properties that we are already committed to on independent grounds. The physicalist theory presented here has some additional advantages. Unlike the most popular version of dispositionalism, it gets by without suspect visual-field properties like Peacocke's red´ (see, for example, Peacocke, this volume, chapter 5). Further, it can accommodate our intuitive judgments of similarity relations among the colors, and the binary/unique distinction. But serious problems -- although not peculiar to physicalism -- remain. Two stand out. First, what makes it the case that visual experience has the representational content that it does? Second, what is the right account of the difference between the various sensory modalities? We are as yet far from satisfactorily answering these questions.[41]
References
Armstrong, D. M. 1978. Universals and Scientific Realism. Cambridge: Cambridge University Press.
Armstrong, D. M. 1989. Universals: An Opinionated Introduction. Boulder, CO: Westview Press.
Block, N. 1990. Inverted Earth. In Philosophical Perspectives 4, ed. J. Tomberlin. Atascadero, CA: Ridgeview.
Block, N. 1996. Mental paint and mental latex. In Philosophical Issues 7, ed. E. Villanueva. Atascadero, CA: Ridgeview.
Block, N. Forthcoming. Mental ink. In Anti-Individualism and Scepticism, ed. M. Hahn and B. Ramberg. Cambridge, MA: MIT Press, forthcoming.
Boghossian, P. A., and J. D. Vellemen. 1989. Colour as a secondary quality. Mind 98, 81-103. Reprinted as chapter 7 of this volume.
Boghossian, P. A., and J. D. Vellemen. 1991. Physicalist theories of color. Philosophical Review 100, 67-106. Reprinted as chapter 8 of this volume.
DeBellis, M. 1991. The representational content of musical experience. Philosophy and Phenomenological Research 51, 303-24.
Dennett, D. 1991. Consciousness Explained. Boston: Little, Brown.
Dretske, F. 1981. Knowledge and the Flow of Information. Cambridge, MA: MIT Press.
Dretske, F. 1988. Explaining Behavior. Cambridge, MA: MIT Press.
Dretske, F. 1995. Naturalizing the Mind. Cambridge, MA: MIT Press.
Dretske, F. 1996. Phenomenal externalism. In Philosophical Issues 7, ed. E. Villanueva. Atascadero, CA: Ridgeview.
Fodor, J. A. 1987. Psychosemantics. Cambridge, MA: MIT Press.
Fodor, J. A. 1990. A theory of content, II: the theory. In his A Theory of Content and Other Essays. Cambridge, MA: MIT Press.
Grandy, R. E. 1989. A modern inquiry into the physical reality of colors. In Mind, Value and Culture: Essays in Honour of E. M. Adams, ed. D. Weissbord. Atascadero, CA: Ridgeview.
Hardin, C, L. 1993. Color for Philosophers: Unweaving the Rainbow (expanded edition). Indianapolis: Hackett.
Harman, G. 1990. The intrinsic quality of experience. In Philosophical Perspectives 4, ed. J. Tomberlin. Atascadero, CA: Ridgeview.
Harman, G. 1996. Explaining objective color in terms of subjective reactions. In Philosophical Issues 7, ed. E. Villanueva. Atascadero, CA: Ridgeview. Reprinted as chapter 13 of this volume.
Hilbert, D. R. 1987. Color and Color Perception: A Study in Anthropocentric Realism. Stanford: CSLI.
Hilbert, D. R. 1992. What is color vision? Philosophical Studies 68, 351-70.
Hurvich, L. M. 1981. Chromatic and achromatic response functions. In his Color Vision. Sunderland, MA: Sinauer Associates. Reprinted as chapter 3 in Readings on Color, vol. 2: The Science of Color.
Johnston, M. 1992. How to speak of the colors. Philosophical Studies 68, 221-63. Reprinted as chapter 9 of this volume.
Johnston, M. 1997. Postscript: visual experience. An appendix to chapter 9 of this volume.
Kay, P., and C. K. McDaniel. 1978. The linguistic significance of the meanings of basic color terms. Language 54, 610-46. Reprinted as chapter 17 in Readings on Color, vol. 2: The Science of Color.
Lewis, D. K. 1983. New work for a theory of universals. Australasian Journal of Philosophy 61, 343-77.
Lycan, W. G. 1987. Consciousness. Cambridge, MA: MIT Press.
Lycan, 1996a. Layered perceptual representation. In Philosophical Issues 7, ed. E. Villanueva. Atascadero, CA: Ridgeview.
Lycan, W. G. 1996b. Consciousness and Experience. Cambridge, MA: MIT Press.
MacAdam, D. L. 1985. The physical basis of color specification. In his Color Measurement: Theme and Variations. New York: Springer-Verlag. Reprinted as chapter 2 in Readings on Color, vol. 2: The Science of Color. References to the latter.
Matthen, M. 1988. Biological functions and perceptual content. Journal of Philosophy 85, 5-27.
Millikan, R. G. 1984. Language, Thought, and Other Biological Categories: New Foundations for Realism. Cambridge, MA: MIT Press.
Nassau, K. 1980. The causes of color. Scientific American (October), 124-54. Reprinted as chapter 1 in Readings on Color, vol. 2: The Science of Color.
Peacocke, C. 1983. Sense and Content: Experience, Thought, and their Relations. Oxford: Oxford University Press.
Peacocke, C. 1984. Colour concepts and colour experience. Synthese 58, 365-82. Reprinted as chapter 5 of this volume.
Peacocke, C. 1992. A Study of Concepts. Cambridge, MA: MIT Press.
Putnam, H. 1975. The meaning of `meaning.' In Language, Mind and Knowledge: Minnesota Studies in the Philosophy of Science 7, ed. K. Gunderson. Minneapolis: University of Minnesota Press.
Shoemaker, S. 1982. The inverted spectrum. Journal of Philosophy 79, 357-81.
Shoemaker S. 1990. Qualities and qualia: what's in the mind? Philosophy and Phenomenological Research 50 (Suppl.), 109-31.
Shoemaker, S. 1991. Qualia and consciousness. Mind 100, 507-24.
Shoemaker, S. 1994a. Self-knowledge and `inner sense.' Lecture III: the phenomenal character of experience. Philosophy and Phenomenological Research 54, 291-314.
Shoemaker, S. 1994b. Phenomenal character. Noûs 28, 21-38. Reprinted as chapter 12 of this volume.
Stalnaker, R. 1989. On what's in the head. In Philosophical Perspectives 3, ed. J. Tomberlin. Atascadero, CA: Ridgeview.
Thompson, E. 1995. Colour Vision. New York: Routledge.
Tolliver, J. T. 1994. Interior colors. Philosophical Topics 22, 411-41.
Tye, M. 1992. Visual qualia and visual content. In The Contents of Experience, ed. T. Crane. Cambridge: Cambridge University Press.
Tye, M. 1994. Qualia, content, and the inverted spectrum. Noûs 28, 159-83.
Tye, M. 1995. Ten Problems of Consciousness: A Representational Theory of the Phenomenal Mind. Cambridge, MA: MIT Press.
Varela, F. J., Thompson, E., and Rosch, E. 1991. The Embodied Mind: Cognitive Science and Human Experience. Cambridge, MA: MIT Press.
Watkins, M. 1995. Dispositionalism in disguise: reply to Averill and Hilbert. Paper delivered at APA Central Division meeting.
Yablo, S. 1993. Is conceivability a guide to possibility? Philosophy and Phenomenological Research 53, 1-42.
[1] The preceding remarks about the content of experience will pass for the purposes of this paper. But they are sketchy, and do not begin to do justice to the many complexities here (for some of these, see Peacocke 1992, chapter 3).
[2] Why "typically?" Because, perhaps, some green-feeling experiences -- for example, the experience of having a green afterimage -- are not green-representing (see Boghossian and Velleman, this volume, chapter 7, section 2.3 of the Introduction, and note 15 below).
[3] Athough relatively uncontroversial this claim is not universally accepted. Tolliver (1994) denies that visual experiences represent objects as having colors. Of course, the claim is also problematic if spectrum inversion is supposed to be a live possibility (but here our assumption that it is not is largely for convenience).
[4] See Hilbert 1987. Defenders of a similar account include Matthen 1988; Grandy 1989; and Tye 1995.
[5] Also for simplicity, we shall ignore the fact that colors vary along dimensions other than hue.
[6] For some remarks about light sources, see Hilbert 1987, pp. 132-4.
[7] Metamerism is not just illumination-relative, but also observer-relative. The phenomenon is treated at greater length in Hilbert 1987; it is argued there that metamerism creates difficulties for some forms of dispositionalism.
[8] It might be claimed that there are colored objects in a possible world where the laws are such that objects reflect and absorb certain ectoplasmic rays, and could not interact with light (and hence do not have reflectances). If so, then colors are not reflectance-types. Perhaps this alleged possibility is even clearly conceivable -- at least in the sense in which philosophers' "zombies" are clearly conceivable. But it would be hasty to remove "alleged." Maybe all we can reasonably conclude is that objects could look colored in a world where objects do not have reflectances -- and we can live with that. For some relevant discussion see Yablo 1993 and Tye 1995, chapter 7.
[9] This will ensure that the hypothesized red-green opponent channel is negative (i.e., biased toward green) and the yellow-blue channel is more or less balanced. See Hardin 1993, chapter 1, and Hurvich 1981. For the graphical representation of an SSR of a typical green paint, see MacAdam 1985, figure 2.1. For present purposes, the details of the psychophysics and physics do not matter.
[10] Of course, even the parts of a dollar bill that look green at arm's length are not uniformly green viewed close up. Thus the parts that look green and so are represented as having SSRGREEN themselves have parts whose reflectances are not of this type. Does this mean that when dollar bills appear at arm's length to have large green regions this is an illusion? No. True, when a region looks uniformly green parts of it are represented as having SSRGREEN, but not arbitrarily small parts. (Cf. Hilbert 1987, chapter 2.)
[11] Taking SSRGREEN to be well-defined is certainly an oversimplification, because of the vagueness of color categories. For an attempt, using fuzzy set theory, to accomodate such vagueness, see Kay and McDaniel 1978.
[12] In Hilbert 1987 (chapters 5, 6) the maximally specific colors are called "maximally determinate colors", "individual colors", or simply "colors", and the fact that this is an extension of everyday talk is not explicitly discussed. For some criticism, see Watkins 1995, esp. pp. 3-4, 11-12.
[13] Loci classici: Shoemaker 1982, Peacocke 1983, Harman 1990 and Block 1990. See also Block 1996, forthcoming; DeBellis 1991; Dennett 1991; Harman, this volume, chapter 13; Shoemaker 1990, 1991, 1994a, this volume, chapter 12; Tye 1992, 1994; Lycan 1987, 1996b; and many of the papers in Philosophical Issues 7, ed. E. Villanueva (Atascadero, CA: Ridgeview, 1996).
[14] See especially pp. XX-YY.
[15] Here are three apparent counterexamples. The first two are directed against the left-to-right part of NECESSITY, the last against the right-to-left part.
First, a case suggested by some remarks of Peacocke's (this volume, chapter 5, pp. XX-YY). If you look through a sheet of green cellophane at a white sheet of paper, rather surprisingly it looks white. Without the cellophane, of course it still looks white. So, it might be argued, the color contents of the two sorts of experiences are the same -- both represent the paper as having the property white -- but there is a clear phenomenological difference between them. There is, as it were, a "green tinge" present in the first sort of experience but not in the second.
However, with the green cellophane in place, the scene before the eyes looks just as if the paper is white and the ambient illumination is green. It is therefore natural to propose that the first experience, but not the second, represents (falsely) that this is the case. Therefore the color content of the two experiences differs.
For the second case, consider the experiences of having, respectively, a red and a green afterimage. They differ, of course, in phenomenology. But, it might be argued, such experiences do not represent anything as having a color, and so they are trivially alike in color content. (Cf. Boghossian and Velleman, this volume, chapter 7, pp. XX-YY.)
However, we think the experience of having a green afterimage represents that there is a green patch some indeterminate distance before the eyes, and hence it is green-representing (and nonveridical). Cf. Harman 1990, p. 40; Tye 1995, pp. 107-8; Lycan 1996a, p. 83. (And in fact, sometimes one mistakes afterimages for colored patches on the surfaces of objects.) So, as in the first case, the color content of the two experiences differs.
For the third case, consider blindsight. Blindsighted patients are, apparently, able to perform above chance when forced to choose the color of an object presented in their blind fields (see Hardin 1993, p. xxx). Two blindsighted patients, one of whom performs above chance (call her "S") and the other at chance, might enjoy visual experiences identical in phenomenology when a tomato is presented in their blind fields. This, is might be argued, is a case of a difference in the color properties represented by visual experience, with no accompanying difference in phenomenology.
However, although this may be a case of different content, it is not a difference in the content of visual experience. The tomato may be represented as having the property red by some part of S's visual system. But since there is certainly no ordinary sense in which the tomato looks red to S (she herself would deny it), the property red does not enter into the content of her visual experience.
[16] Someone who is persuaded to deny NECESSITY on the basis of either the inverted spectrum argument or one of the first two cases given in note 15 above will think that there are (possible) green-feeling experiences that are not green-representing. So she owes us an account of what is common to all and only green-feeling experiences. It cannot be color content, so what is it? It is worth briefly examining Peacocke and Shoemaker's answers to this question.
First, Peacocke's answer: all green-feeling experiences present the "sensational property" green´ ("green-prime") to the subject. Green´ is, of course, not the property green, and not a property objects are represented as having. Rather, it is supposed to be a property of "regions of the visual field" -- a property, that is, of something like sense-data. (See Peacocke 1983, chapter 1, 1992, pp. 7-8, and this volume, chapter 5.)
Although we shall not argue for this here, we think that once sensational properties like green´ are admitted, an error theory of color experience is inescapable: the common person mistakenly takes green´ to be a property of gooseberries and cucumbers (for precisely this view, see Boghossian and Velleman, this volume, chapters 7, 8).
Shoemaker's ingenious answer (this volume, chapter 12) is that while all green-feeling experiences do not share a color content, they all represent objects as having a "phenomenal property," which we may call "phenomenal-green." Phenomenal-green (not to be confused with green) is the property of "producing in a viewer" green-feeling experiences, and is thus a property things do not have when they are not being perceived (pp. XX). But Shoemaker's view has, like Peacocke's, the disadvantage that it leads to an error theory, or so it seems to us. According to Shoemaker, when Invert and Nonvert look at a raspberry, their experiences each represent it as having two properties: phenomenal-green and red (Invert) and phenomenal-red and red (Nonvert). If Shoemaker is right that experience represents objects as having phenomenal-colors, then we think that the common person mistakenly takes objects to have these properties when they are not being perceived.
[17] In fact, we think that Fred's case is simply an especially vivid way of making points that could be made using the standard inverted spectrum case, and is therefore a ladder that may be kicked away. But it does not seem fruitful to insist on this, because it seems most unlikely that our opponents would agree.
[18] Fred's plight might be described as a case of partial intrapersonal spectrum inversion on Alternately Inverting Earth (cf. Block 1990). We should emphasize that we are not supposing that Fred in principle could not tell on the basis of his visual experience that something is awry. Given certain assumptions about his environment and the asymmetry of color space, perhaps he could. All we are supposing is that (perhaps due to limitations on his part) he is not able to notice any difference.
[19] Of course, the conclusion that Invert sees the true colors of objects is not entailed by the premise that color content is extrinsic. What is true, rather, is this. Most, maybe all, plausible theories of extrinsic color content entail that Invert (or Invert as he appears in a more intricate example) sees the true colors of objects.
[20] It might be objected that this is not right, because Fred and Invert differ in a crucial respect (here we are indebted to Robert Stalnaker). Fred remembers that some objects look the same (in respect of color) to him on Tuesday as other objects did on Monday (in Shoemaker's "qualitative sense" of "looks the same" -- see Shoemaker 1982). In particular, suppose that Fred is looking at a strawberry on Tuesday and recalls that it looks the same as a raspberry he saw on Monday. Now if our description of Fred's case is correct, the raspberry was represented by Fred's experience on Monday as green, while the strawberry is represented as red -- yet he remembers that they look the same! And this, it might be thought, is not plausible. (Compare Invert's case: he remembers that a raspberry looked the same to him yesterday as a strawberry does on today, but his experiences represent them as having the same color.)
We reply as follows. Cases where someone undergoes spectrum inversion and, after a while, sees the true colors of objects as before, are taken, by the proponents of the inverted spectrum argument, to be clearly possible. (Indeed, more so than cases of spectrum inversion from birth.) Thus the subject remembers that some objects (e.g. gooseberries) looked the same to him before the inversion as others (e.g. raspberries) do after the inversion, but even so gooseberries were represented as green, whereas raspberries are represented as red. If this is not problematic, why suppose Fred's case is?
[21] It might be replied that although it appears to Fred that the world has not changed, he mistakenly believes that it appears to him that the world has changed. That is, Fred is the victim of an illusion with respect to his experience: he believes that his visual experiences differ in content, but in fact there is no difference. But this reply does not work, for at least two reasons. First, we may suppose that Fred, perhaps like many of us in normal circumstances, has no beliefs about his visual experience -- he just has beliefs about the scene before his eyes. Second, we may alternatively suppose that Fred is apprised of his role in the Gedankenexperiment, and is a proponent of the inverted spectrum argument. He will then believe that his visual experiences are the same in content, but the world will still appear to him to change.
[22] Another reply -- requiring some stage-setting -- is this. Return to Invert and Nonvert, both looking at a gooseberry. A proponent of the inverted spectrum argument will usually say that this is not just a case of same properties represented, but a case of same content simpliciter. Call this the "Same Content Claim" (see, for example, Shoemaker 1982, Block 1990). But there is another way of taking the inverted spectrum, equally inhospitable to NECESSITY, which we will call the "Fregean Response".
According to the Fregean Response, two visual experiences may differ in content despite representing objects as having the same properties. Visual experiences, on this account, represent properties under various "modes of presentation," akin to Fregean senses. And these modes of presentation are supposed to enter into the content of the experience.
The Fregean Response and the Same Content Claim both agree that Invert and Nonvert's experiences are the same in respect of what we are calling "color content": they both represent the raspberry as having the property red. But the Fregean Response, unlike the Same Content Claim, says that the experiences differ in their visual modes of presentation of this property, and thus in their content.
Now go back to Fred and the red raspberry. According to the Same Content Claim, the change in Fred's experience is not a change in content -- it is solely a change in phenomenology. We complained that it is a change in content. So far, so good.
However, it might be objected that our complaint is of no force against the Fregean Response. According to it, the content of Fred's experience does change. When he takes his first look, the raspberry is represented as having the property red under one mode of presentation, and when he takes his second, the raspberry is represented as having this same property under a different mode of presentation.
But the Fregean Response does not do justice to the fact that the raspberry appears to Fred to change. The raspberry changes only if it gains or loses a property, and so the raspberry will appear to change only if it appears to gain or lose a property. If the difference between Fred's experiences were simply a difference in modes of presentation, then the raspberry would not appear to gain or lose a property, and so would not appear to change. That is, if the Fregean Response were right, then although the way the raspberry appears would change, the raspberry itself would not appear to change.
To see the difference, consider a case where modes of presentation might do useful work: distinguishing between sensory modalities (cf. Shoemaker 1990). Arguably, the very same property -- shape -- can be represented by both tactile and visual experiences. If that's right, it would be natural to appeal to modes of presentation to give a representational account of the phenomenological difference between seeing that an object is square and feeling that it is. To switch to a hypothetical example, suppose that Fred can taste colors. Red things have a distinctive taste that green things lack, and so on. The property red (we are pretending) is a property that certain of Fred's visual and gustatory experiences both represent raspberries as having. The experiences are different, it might be claimed, because they differ in content at the level of sense rather than reference; that is, Fred's visual and gustatory modalities represent the same property -- red -- under different modes of presentation. Here the appeal to modes of presentation, whatever its other defects, does not suffer from the problem just raised for the Fregean Response. When Fred successively sees and tastes a raspberry, the way the raspberry appears changes, but the raspberry itself does not appear to change.
[23] Sometimes philosophers use "narrow content" to refer to what we have here called "intrinsic content". But "narrow content" is used ambiguously in a way that invites confusion. Some say that while Oscar and Twoscar do not share a belief with the content that water is wet, they do share some other mental state: a mental state that determines, together with the environmental facts, whether the subject believes that water is wet, or whether she believes that twater is wet. Such a state -- the organismic contribution to believing that water is wet (and to believing that twater is wet) -- is supposed to have "narrow content" (see, for example, Fodor 1987). Narrow content in this sense is at best some etiolated kind of content and at worst unintelligible (for some classic objections see Stalnaker 1989). This sense of "narrow content" is not what we mean by "intrinsic content": the latter is just familiar propositional content that is necessarily shared between duplicates.
[24] We should record that one of us (DH) prefers to deny the first premise, while the other (AB) prefers to deny the third.
[25] Hilbert 1987 implicitly assumes a correlational theory of content in the style of Dretske 1981, and Hilbert 1992 explicitly assumes a teleological theory in the style of Millikan 1984. These two theories do exclude intrinsic content.
[26] See Peacocke 1992, pp. 74-6, from which the example is taken.
[27] These definitions are inevitably somewhat stipulative. For instance, we might just as well have said that x and y are perfectly similar relative to a family of properties {P1,..., Pn} just in case x and y either both have or both lack each Pi. And we might just as well have said that x is more similar to y than to z, relative to a family of properties {P1,..., Pn} just in case x and y share some Pi that z lacks, and if x and z have some Pj, then y has Pj.. But for the argument to follow, the (second) definition given in the text is the one we want.
[28] We are here assuming that properties are "abundant" in Lewis's (1983) sense. To every set of possible things, no matter how diverse, each thing paired with a possible world it inhabits, there corresponds a property had by all and only those things in those worlds. Assuming, as Lewis holds, that possible individuals inhabit only one world, we can put this more cleanly as: to every set of possible things, no matter how diverse, there corresponds a property had by all and only those things.
[29] For a defense of natural similarity (although not under this name) see Lewis 1983; and Armstrong 1978, 1989. Natural similarity is not usually left as primitive: Armstrong, for instance, analyzes simple cases of it as the sharing of certain special sorts of properties -- universals -- which are wholly present wherever they are instantiated.
[30] Cf. Johnston, this volume, chapter 9, pp. XX; and Boghossian and Velleman, chapter 8, pp. XX.
[31] See, for example, Armstrong 1989, p. 33.
[32] See MacAdam 1985, figures 2.1, 2.2, and 2.6.
Hilbert 1987 (chapter 6) can be understood as arguing that physicalism can accomodate SIMILARITY (although perhaps not our knowledge of it). There colors are identifed with certain triples of integrated reflectances. It is claimed that a certain space of such triples, with a physically motivated metric, is roughly isomorphic to color similarity space. But this is not right, or not right enough: the space of triples provides only a very loose approximation to similarity relations among the colors. See Thompson 1995, chapter 3, for detailed discussion.
[33] Here (although not in the statement of NECESSITY) "possible visual experience" should be understood to be restricted to visual experiences that can be produced by the human visual system (as it actually is). On our view, we cannot rule out the possibility that some strange kinds of visual systems can produce an experience that represents three objects x, y, and z to be scarlet, crimson, and lemon yellow, but does not represent (for example) x and y to be red. (Although, of course, every scarlet object is red.) Dropping the restriction to the human visual system would therefore imply that the defined three-place relation does not hold between any color properties.
[34] See Armstrong 1978, chapters 21, 22; 1989, pp. 103-7.
[35] If we wish to include the achromatic colors the list of unique hues should include black and white. Brown is a puzzling case (see Hardin 1993, p. 141). These complications do not affect our argument and will be ignored in what follows.
[36] See Readings on Color, vol. 2: The Science of Color.
[37] For endorsements of this complaint, see Varela et al., p. 166; Thompson 1995, pp. 135-9. (Thompson's endorsement is somewhat qualified: he thinks the argument shows that colors are not "nonrelational" properties.)
[38] Pace Tye 1995, p. 148.
[39] Hardin's official demand is that "those physical complexes must admit of a division into unique and binary complexes." On a uncharitable reading, this is easy to meet: just stipulate that the physical properties to be identified with red, green, yellow, and blue are to count as unique, and the rest binary. But we take it that Hardin thinks that (if physicalism is to be saved) the division must be physically motivated.
[40] Our response to this objection also provides an account of the opponent structure of the hues. Red is opposed to green, and blue is opposed to yellow, because no object is represented by the human visual system simultaneously as having reddishness and greenishness, or yellowishness and bluishness. But we are not offering any explanation of this fact (see the end of 3.3 above).
[41] We are particularly indebted for comments on early drafts and much discussion to Ned Hall, Katie Hilbert, Jim Pryor, Judith Thomson, Robert Stalnaker, and Ralph Wedgwood. For comments on later versions, thanks to Justin Broackes, Fiona Cowie, Mark Crimmins, Daniel Stoljar, Sarah Stroud, and Mark Johnston.