lecture9

13.021 - Marine Hydrodynamics, Fall 2003

Lecture 9

13.021 - Marine Hydrodynamics
Lecture 9

Vorticity Equation

Return to viscous incompressible flow.

N-S equation:

$\displaystyle \frac{\partial \vec{v} }{\partial t} + \left(\vec{v} \cdot \nabl... ...{v} = - \nabla \left( {\frac{p}{\rho } + gy} \right) + \nu \nabla ^2\vec{v}$

Then,

$\displaystyle \nabla \times \left(\mbox{N-S equation}\right)\buildrel \over \lo... ...a \times \left(\vec{v} \cdot \nabla\right)\vec{v} = \nu \nabla^2\vec{\omega }$

since $\nabla \times \nabla \phi = 0$ for any $\phi$ (conservative forces)

Now consider the vector identities:

$\displaystyle (\vec{v} \cdot \nabla)\vec{v}$	$\displaystyle = \frac{1}{2}\nabla \left( \vec{v} \cdot \vec{v} \right) - \vec{v} \times \left( {\nabla \times \vec{v} } \right)$
	$\displaystyle = \nabla \left( {\frac{v^2}{2}} \right) -\vec{v} \times \vec{\omega }$ where $\displaystyle v^2 \equiv \left\vert\vec{v} \right\vert^2 = \vec{v} \cdot \vec{v}$
$\displaystyle \nabla \times \left({ \vec{v}} \cdot \nabla \right) \vec{v}$	$\displaystyle = \nabla \times \nabla \left( {\frac{v^2}{2}} \right) - \nabla \t... ...vec{\omega } \right) = \nabla \times \left(\vec{\omega } \times \vec{v} \right)$
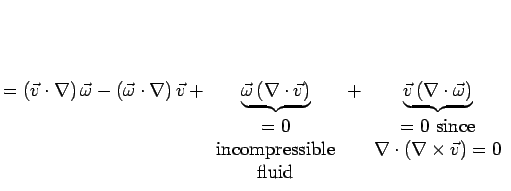	$\displaystyle = \left( \vec {v} \cdot \nabla \right)\vec{\omega} - \left( { \v... ...d{array}}{\underbrace{ \vec {v} \left( {\nabla \cdot \vec{\omega }} \right)}}$

Therefore,

$\displaystyle \frac{\partial \vec{\omega } }{\partial t}$	$\displaystyle + \left(\vec{v} \cdot \nabla \right) \vec{\omega } = \left(\vec{\omega } \cdot \nabla \right) \vec{v} +\nu\nabla^{2} \vec{\omega }$
or
$\displaystyle \frac{D \vec{\omega } }{Dt}$	$\displaystyle = \left(\vec{\omega } \cdot \nabla \right) \vec{v} + \underbrace{\nu \nabla ^2 \vec{\omega} }_{\mbox{diffusion}}$

Kelvin's Theorem revisited.

If $\nu \equiv 0,$ then $\frac{D \vec{\omega } }{Dt} = \left(\vec{\omega } \cdot \nabla \right) \vec{v}$ , so if $\vec{\omega } \equiv 0$ everywhere at one time, $\vec{\omega } \equiv 0$ always.
$\nu$ can be thought of as diffusivity of (momentum) and vorticity, i.e., $\vec{\omega }$ once generated (on boundaries only) will spread/diffuse in space if $\nu$ is present.

$\begin{figure} \begin{center} \epsfig{file=lfig91.eps,height=1.65in,width=5.5in,clip=} \end{center} \end{figure}$
Diffusion of vorticity is analogous to the heat equation: $\frac{\partial T}{\partial t} = K\nabla ^2T$ , where K is the heat diffusivity

Also since $\nu \sim$ 1 or 2 mm $^{2}$ /s, in 1 second, diffusion distance $\sim O\left( {\sqrt {\nu t} } \right)\sim O\left( {mm} \right)$ , whereas diffusion time $\sim O\left( {{L^2} \mathord{\left/ {\vphantom {{L^2} \nu }} \right. \kern-\nulldelimiterspace} \nu } \right)$ . So for a diffusion distance of L = 1cm, the necessary diffusion time needed is O(10)sec.
For 2D, $\vec{v} = \left( {u,v,0} \right)$ and $\frac{\partial }{\partial z} \equiv 0$ . So, $\vec{\omega } = \nabla \times \vec{v}$ is $\bot$ to $\vec{v}$ (parallel to z-axis).
Then,

$\displaystyle \left( \vec{\omega } \cdot \nabla \right) \vec{v} = \left( {\unde... ...a _z \underbrace {\frac{\partial }{\partial z}}_0} \right) \vec{v} \equiv 0,$

so in 2D we have

$\displaystyle \frac{D \vec{\omega } }{Dt} = \nu \nabla^2 \vec{\omega }.$

If $\nu$ = 0, $\frac{D \vec{\omega } }{Dt} = 0$ , i.e. in 2D, following a particle, the angular velocity is conserved. Reason: in 2D, the length of a vortex tube cannot change due to continuity.
For 3D,

$\displaystyle \frac{D\omega _i }{Dt} = \underbrace{\omega _j \frac{\partial v_i... ...ace {\nu \frac{\partial ^2\omega _i }{\partial x_j \partial x_j }}_{diffusion}$

For example:

$\displaystyle \frac{D\omega _2 }{Dt} = \underbrace {\omega _1 \frac{\partial u_... ...ac{\partial u_2 }{\partial x_3 }}_{\mbox{vortex turning}} + \mbox{diffusion}$

$\begin{figure} \begin{center} \epsfig{file=lfig92.eps,height=2in,clip=} \end{center} \end{figure}$

Example: Pile on a River

$\begin{figure} \begin{center} \epsfig{file=lfig93.eps,height=1.5in,clip=} \end{center} \end{figure}$

What really happens as the length of the vortex tube L increases?

IFCF (Ideal fluid under the influence of conservative forces) is no longer a valid assumption.

Why?

Ideal flow assumption implies that the inertia forces are much larger than the viscous effects (Reynolds number).

$\displaystyle R_e \sim \frac{UL}{\nu }$ Length increases $\displaystyle \Rightarrow$ diameter becomes really small $\displaystyle \Rightarrow \quad R_{e}$ is not that big after all. $\displaystyle$

Therefore IFCF is no longer valid.

3.3 Potential Flow - ideal (inviscid and incompressible) and irrotational flow

If $\vec{\omega } \equiv 0$ at some time , then $\vec{\omega } \equiv 0$ always for ideal flow under conservative body forces by Kelvin's theorem. Given a vector field $\vec{v}$ for which $\vec{\omega } = \nabla \times \vec{v} \equiv 0$ , then there exists a potential function (scalar) - the velocity potential - denoted as $\phi$ , for which

$\displaystyle \vec{v} = \nabla \phi$

Note that $\vec{\omega } = \nabla \times \vec{v} = \nabla \times \nabla \phi \equiv 0$ for any $\phi$ , so irrotational flow guaranteed automatically. At a point $\vec{x}$ and time , the velocity vector $\vec{v}(\vec{x},t)$ in cartesian coordinates in terms of the potential function $\phi(\vec{x},t)$ is given by

$\displaystyle \vec{v} \left(\vec{x} ,t \right) = \nabla \phi \left(\vec{x} ,t \... ...x},\frac{\partial \phi }{\partial y},\frac{\partial \phi}{\partial z} \right)$

$\begin{figure} \begin{center} \epsfig{file=lfig94.eps,height=2in,clip=} \end{center} \end{figure}$

The velocity vector $\vec{v}$ is the gradient of the potential function $\phi$ , so it always points towards higher values of the potential function.

Governing Equations:

Continuity:

$\displaystyle \nabla \cdot \vec{v} = 0 = \nabla \cdot \nabla \phi \Rightarrow \nabla ^2\phi = 0$

Number of unknowns $\to \quad \phi$

Number of equations $\to \quad \nabla ^2\phi = 0$

Therefore the problem is closed. $\phi$ and (pressure) are decoupled. $\phi$ can be solved independently first, and after it is obtained, the pressure is evaluated.

$\displaystyle p = f\left( \vec{v} \right) = f\left( \nabla \phi \right) \quad \to$ Solve for $\displaystyle \phi ,$ then find pressure.

3.4 Bernoulli equation for potential flow (steady or unsteady)

Euler eq:

$\displaystyle \frac{\partial \vec{v} }{\partial t} + \nabla \left( \frac{v^2}{2... ...) - \vec{v} \times \vec{\omega } = - \nabla \left( \frac{p}{\rho } + gy \right)$

Substitute $\vec{v} = \nabla \phi$ into the Euler's equation above, which gives:

$\displaystyle \nabla \left( {\frac{\partial \phi }{\partial t}} \right) + \nabl... ...\phi } \right\vert^2} \right) = - \nabla \left( {\frac{p}{\rho } + gy} \right)$

$\displaystyle \nabla \left\{ {\frac{\partial \phi }{\partial t} + \frac{1}{2}\left\vert {\nabla \phi } \right\vert^2 + \frac{p}{\rho } + gy} \right\} = 0,$

which implies that

$\displaystyle \frac{\partial \phi }{\partial t} + \frac{1}{2}\left\vert {\nabla \phi } \right\vert^2 + \frac{p}{\rho } + gy = f(t)$

everywhere in the fluid for unsteady, potential flow. The equation above can be written as

$\displaystyle p = - \rho \left[ {\frac{\partial \phi }{\partial t} + \frac{1}{2}\left\vert {\nabla \phi } \right\vert^2 + gy} \right] + F(t)$

which is the Bernoulli equation for unsteady or steady potential flow.

Summary: Bernoulli equation for ideal flow.

Steady rotational or irrotational flow along streamline.

$\displaystyle p = - \rho \left( {\frac{1}{2}v^2 + gy} \right) + C(\psi )$
Unsteady or steady irrotational flow everywhere in the fluid.

$\displaystyle p = - \rho \left( {\frac{\partial \phi }{\partial t} + \frac{1}{2}\left\vert {\nabla \phi } \right\vert^2 + gy} \right) + F(t)$
For hydrostatics, $\vec{v} \equiv 0,\frac{\partial }{\partial t} = 0$ .

$\displaystyle p = - \rho gy + c \leftarrow$ hydrostatic pressure (Archimedes' principle)
Steady and no gravity effect ( $\frac{\partial }{\partial t} = 0,g \equiv 0$ )

$\displaystyle p = - \frac{\rho v^2}{2} + c = - \frac{\rho }{2}\left\vert {\nabla \phi } \right\vert^2 + c \leftarrow$ Venturi pressure (created by velocity)
Inertial, acceleration effect

$\begin{displaymath}\begin{array}{c} p\sim - \rho \frac{\partial \phi }{\partia... ...\frac{\partial }{\partial t} \vec{v} + \cdots \\ \end{array}\end{displaymath}$

$\begin{figure} \begin{center} \epsfig{file=lfig95.eps,height=2in,clip=} \end{center} \end{figure}$

3.5 - Boundary Conditions

KBC on an impervious boundary

$\displaystyle \underbrace { \vec{v} \cdot \hat {n}}_{\hat {n} \cdot \nabla \ph... ... boundary\ } \Rightarrow \frac{\partial \phi }{\partial n} = U_n \mbox{ given}$
DBC: specify pressure at the boundary, i.e.,

$\displaystyle - \rho \left( {\frac{\partial \phi }{\partial t} + \frac{1}{2}\left\vert {\nabla \phi } \right\vert^2 + gy} \right) = \mbox{given}$

Note: On a free-surface $p = p_{atm}$ .

3.6 - Stream function

continuity: $\nabla \cdot\vec{v} = 0$ ; irrotationality: $\nabla \times \vec{v} = \vec{\omega } = 0$
velocity potential: $\vec{v} = \nabla \phi$ , then $\nabla \times \vec{v} = \nabla \times \left( {\nabla \phi } \right) \equiv 0$ for any $\phi,$ i.e. irrotationality is satisfied automatically. Required for continuity:

$\displaystyle \nabla \cdot \vec{v} = \nabla ^2\phi = 0$
Stream function $\vec{\psi }$ defined by

$\displaystyle \vec{v} = \nabla \times \vec{\psi }$

Then $\nabla \cdot \vec{v} = \nabla \cdot \left( {\nabla \times \vec{\psi } } \right) \equiv 0$ for any $\vec{\psi },$ i.e. satisfies continuity automatically.
Required for irrotationality:

$\displaystyle \nabla \times \vec{v} = 0 \Rightarrow \nabla \times \left( \nabla... ...race{\nabla \left( \nabla \cdot \vec {\psi } \right) - \nabla^2\vec{\psi}}} = 0$ (1)
For 2D and axisymmetric flows, $\vec{\psi }$ is a scalar $\psi$ (so stream functions are more useful for 2D and axisymmetric flows).

For 2D flow: $\vec{v} = \left( {u,v,0} \right)$ and $\frac{\partial }{\partial z} \equiv 0$ .

$\displaystyle \vec{v} = \nabla \times \vec{\psi } = \left\vert {{\begin{array}{... ...}{\partial x}\psi _y - \frac{\partial }{\partial y}\psi _x } \right)\hat {k}$

Set $\psi _x = \psi _y \equiv 0$ and $\psi _z = \psi$ , then $u = \frac{\partial \psi }{\partial y}; v = - \frac{\partial \psi }{\partial x}$

So, for 2D:

$\displaystyle \nabla \cdot \vec{\psi } = \frac{\partial }{\partial x}\psi _x + \frac{\partial}{\partial y}\psi _y + \frac{\partial }{\partial z}\psi _z \equiv 0$

Then, from the irrotationality (see (1)) $\Rightarrow \quad \nabla ^2\psi = 0$ and $\psi$ satisfies Laplace's equation.
2D polar coordinates: $\vec{v} = \left( {v_r ,v_{_\theta }} \right)$ and $\frac{\partial }{\partial z} \equiv 0$ .

$\begin{figure} \begin{center} \epsfig{file=lfig96.eps,height=2in,clip=} \end{center} \end{figure}$

$\displaystyle \vec{v} = \nabla \times \vec{\psi } = \frac{1}{r}\left\vert {{\b... ...heta - \frac{\partial }{\partial \theta }\psi _r } \right)}^{v_z }\hat {e}_z$

Again let $\psi _r = \psi _\theta \equiv 0$ and $\psi _z = \psi$ , then $v_r = \frac{1}{r}\frac{\partial \psi }{\partial \theta }$ and $v_\theta = - \frac{\partial \psi }{\partial r}$ .
For 3D but axisymmetric flows, $\vec{\psi }$ also reduces to $\psi$ (read JNN 4.6 for details).
Physical Meaning of $\psi$ .
In 2D: $u = \frac{\partial \psi }{\partial y}$ and $v = - \frac{\partial \psi }{\partial x}$ .

We define

$\displaystyle \psi(\vec{x},t) = \psi(\vec{x}_{0},t)+\underset{\begin{array}{c} ... ...v}\cdot\hat{n}dl}} = \psi(\vec{x}_{0},t)+\int_{\vec{x}_{0}}^{\vec{x}}(udy-vdx)$

$\begin{figure} \begin{center} \epsfig{file=lfig97.eps,height=2in,clip=} \end{center} \end{figure}$

For $\psi$ to be single-valued, $\int$ must be path independent.

$\displaystyle \int\limits_C { = \int\limits_{C'} }$ or $\displaystyle \int\limits_C { - \int\limits_{C'} { = 0} } \buildrel \over \lon... ...nt\!\!\!\int\limits_S \underbrace{ \nabla \cdot \vec{v} }_{continuity}ds = 0$

Therefore, $\psi$ is unique because of continuity.

Let $\vec{x} _1, \vec{x} _2$ be two points on a given streamline ( $\vec{v} \cdot \hat {n} = 0$ on streamline)

$\begin{figure} \begin{center} \epsfig{file=lfig98.eps,height=2in,clip=} \end{center} \end{figure}$

$\displaystyle \underbrace {\psi \left( { \vec{x} _2 } \right)}_{\psi _{_2 } } ... ...\begin{array}{c} = 0 \\ \mbox{ along } \\ \mbox{ streamline} \end{array} }dl }$

Therefore, $\psi _{1} = \psi_{2}$ ,i.e., $\psi$ is a constant along any streamline. For example, on an impervious stationary body $\vec{v} \cdot \hat {n} = 0$ , so $\psi$ = constant on the body is the appropriate boundary condition. If the body is moving $\vec{v} \cdot \hat {n} = U_n$

$\displaystyle \psi = \psi _0 + \int {\underbrace {U_n }_{given}dl }$ on the boddy

$\begin{figure} \begin{center} \epsfig{file=lfig99.eps,height=2in,clip=} \end{center} \end{figure}$

Flux $\Delta \psi = - v\Delta x = u\Delta y$ . Therefore, $u = \frac{\partial \psi }{\partial y}$ and $v = - \frac{\partial \psi }{\partial x}$

$\begin{figure} \begin{center} \epsfig{file=lfig910.eps,height=2.5in,clip=} \end{center} \end{figure}$

Summary: Potential formulation vs. Stream-function formulation for ideal flows

table

	potential	stream-function
definition	$\vec{v} = \nabla \phi$	$\vec{v} = \nabla \times \vec {\psi }$
continuity $\nabla \cdot\vec{v} = 0$	$\nabla ^2\phi = 0$	automatically satisfied
irrotationality $\nabla \times \vec{v} = 0$	automatically satisfied	$\nabla \times \left( {\nabla \times \vec {\psi } } \right) = \nabla \left( {\nabla \cdot \vec {\psi } } \right) - \nabla ^2 \vec {\psi } = 0$
in 2D $w = 0,\frac{\partial }{\partial z} = 0$	$\nabla ^2\phi = 0$	$\psi \equiv \psi _z : \nabla ^2 y\vec {\psi } = 0$
Cartesian (x, y)	$\begin{displaymath}\begin{array}{l} u = \mbox{ }\frac{\partial \phi }{\partial... ...tial y} = - \frac{\partial \psi }{\partial x} \\ \end{array}\end{displaymath}$	Cauchy-Riemann equations for ( $\phi$ , $\psi )$ = (real, imaginary) part of an analytic complex function of z = x +iy
Polar (r, $\theta )$	$\begin{displaymath}\begin{array}{l} v_r = \mbox{ }\frac{\partial \phi }{\parti... ...theta } = - \frac{\partial \psi }{\partial r} \\ \end{array}\end{displaymath}$

For irrotational flow	use	$\phi$
For incompressible flow	use	$\psi$
For both flows	use	$\phi$ or $\psi$

Given $\phi$ or $\psi$ for 2D flow, use Cauchy-Riemann equations to find the other:

For example: $\phi$ = xy $\psi$ = ?

$\displaystyle \left. {\begin{array}{l} \frac{\partial \phi }{\partial \mbox{x}... ...2 + f_2 (y) \\ \end{array}} \right\} \psi = \frac{1}{2}(y^2 - x^2) + const$

Karl P Burr 2003-09-02