Stream + source/sink pair: Rankine closed bodies

To have a closed body, a necessary condition is to have $\sum {m_{\mbox{in body}} } = 0$

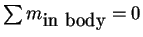
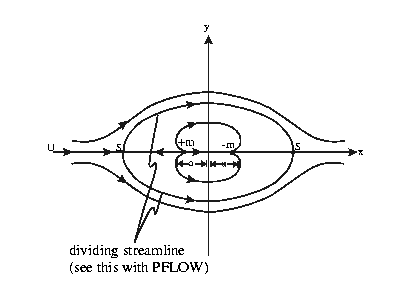
2D Rankine ovoid:

$$\phi = Ux + \frac{m}{2\pi }\left( {\ell n\sqrt {\left( {x + a... ... y^2} - \ell n\sqrt {\left( {x - a} \right)^2 + y^2} } \right)$$

Stagnation Points for 2D Rankine ovoid

3D Rankine ovoid:

$$\phi = Ux - \frac{m}{4\pi }\left[ {\frac{1}{\sqrt {\left( {x ... ...rac{1}{\sqrt {\left( {x - a} \right)^2 + y^2 + z^2} }} \right]$$

1.

Question: Find the stagnation points for the 3D Rankine ovoid.

(a)

Hint: derive the velocity vector $\vec{v} = 0$.

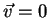(b)

Hint: solve 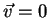$\vec{v} = 0$ for $(x,y,z)$.

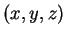2.

Question: Find the radius 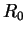$R_{0}$ of the body.

(a)

Hint: mass flux at the half body equals $m$.

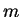Keyword Search