Repulsive Forces?

Abraham-Lorentz+Casimir electron model

H.B.G. Casimir, Physics 19, 846 (1956)

Casimir's "mousetrap" to catch the fine structure constant:

Model the electron as a spherical conducting shell of radius R , with a uniform charge e .

Balance the repulsive Coulomb energy, with the (presumed attractive) Casimir energy:

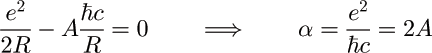
T.H. Boyer, Phys. Rev. 174, 1764 (1968)

"Quantum Electromagnetic Zero-Point Energy of a Conducting Spherical Shell and the Casimir Model for a Charged Particle"

2A = - 0.09235  is negative!  [also obtained by R. Balian and B. Duplantier (1977)]

Does this imply a repulisve force?

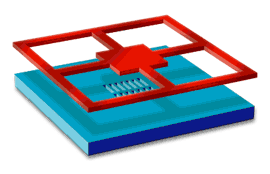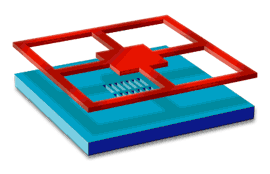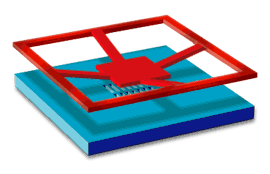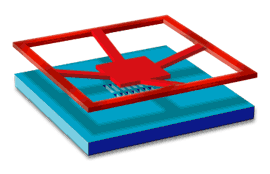
Schematic illustration of experiment to measure repulsive vacuum forces using MEMS oscillator

from Quantum Fields LLC

S.G. Mamaev and N.N. Trunov, Sov. Phys. J. (USA) 22, 51 (1979)

There is a corresponding "repulsive energy" for a parallelopiped

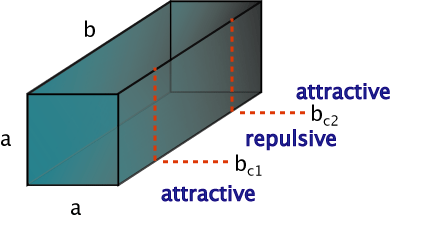
The Casimir Piston

A piston geometry provides an experimentally realizable configuration.

R.M. Cavalcanti, Phys. Rev. D 69, 065015 (2004) (Dirichlet and 2d)

M.P. Hertzberg, R.L. Jaffe, M. Kardar, and A. Scardicchio, Phys. Rev. Lett. 95, 250402 (2005).

When contributions from both sides of the partition are included,

the net force is found to be attractive.

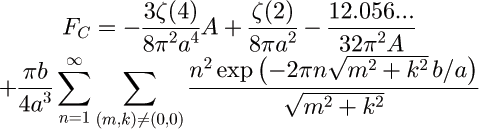
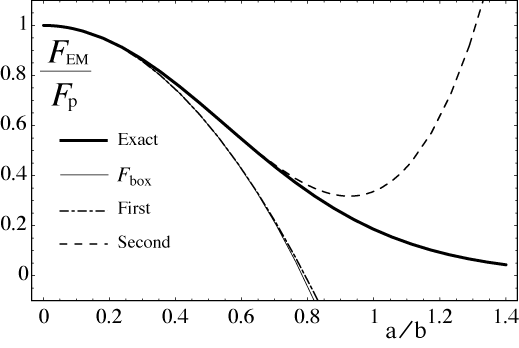
The expansion in height can be generalized to pistons of arbitrary cross-section

[using the density of states formulas of  R. Balian and C. Bloch (1970) ]

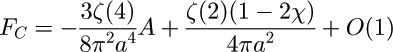
where  χ depends on the 2-dimensional cross-section as

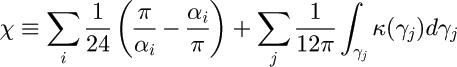
(α is the interior angle of each corner, and κ is the curvature of smooth sections)