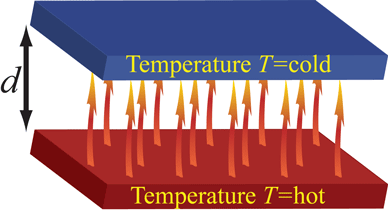
Thermal Disequilibrium
![]() Radiation Pressure from a hot-plate can in principle counteract Casimir attraction
Radiation Pressure from a hot-plate can in principle counteract Casimir attraction
 cf.
cf. 
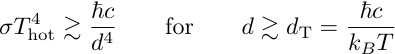
Around room temperature, this is for distances exceeding 7 microns; somewhat large for practical applications,
while at short separations "near-field effects" due to evanescent waves lead to non-classical behavior.
![]() Breaking the law, at the nanoscale (MIT news, July 29, 2009)
Breaking the law, at the nanoscale (MIT news, July 29, 2009)
"Surface Phonon Polaritons Mediated Energy Transfer between Nanoscale Gaps,"
S. Shen, A. Narayanaswamy, & G. Chen, Nano Lett. 9, 2909 (2009)

Heat transfer between plates diverges at short distances due to evanescent waves (tunneling).
![]() A generalized scattering approach enables computation of Casimir forces, as well as radiation and heat transfer.
A generalized scattering approach enables computation of Casimir forces, as well as radiation and heat transfer.
"Nonequilibrium Electromagnetic Fluctuations: Heat Transfer and Interactions,"
M. Krüger, T. Emig, and M. Kardar, Phys. Rev. Lett. 106, 210404 (2011)
Rytov (1959):  "Fluctuational QED"
"Fluctuational QED"
Fluctuating currents in each object are related to its temperature by a fluctuation-dissipation condition:
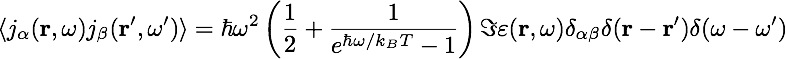
The EM field due to thermal fluctuations of one object is related to overall Green's function by:

The overall fluctuations with many objects at different temperatures is then given by:
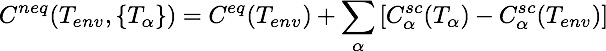
From EM correlations follow the stress tensor and the Poynting vector, hence forces and radiation.
![]() Heat Transfer from a plate to a sphere (and other objects at proximity):
Heat Transfer from a plate to a sphere (and other objects at proximity):
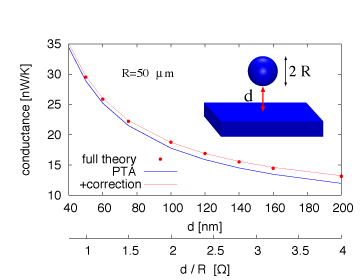
Due to its "divergence" heat transfer is dominated by points of close proximity.
A "Proximity Transfer Approximation (PTA)" with "gradient correction" can by used to compute results for arbitrary smooth shapes at close proximity.
![]() Emission from a single object (Sphere or Cylinder):
Emission from a single object (Sphere or Cylinder):

Emission is proportional to volume for small objects, crossing over to surface proportionality.
Emission from a cylinder is polarized (also switching as a function of size)
"Thermalization of Heat radiation of an Individual Object Thinner than the Thermal Wavelength,"
C. Wuttke and A. Rauschenbeutel, Phys. Rev. Lett. 111, 024301 (2013)
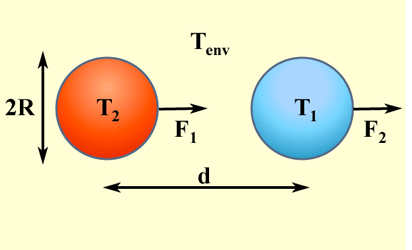
![]() Consider forces between two spheres at different temperatures:
Consider forces between two spheres at different temperatures:
"Non-equilibrium Casimir forces: Spheres and sphere-plate,"
M. Krüger, T. Emig,G. Bimonte and M. Kardar, Europhys. Lett. 95, 21002 (2011)
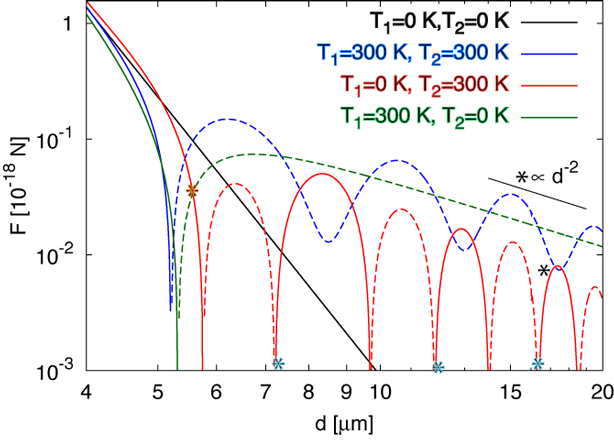
Whereas the nonequilibrium force falls off as 1/d6, the non-equilibrium force decays as 1/d2. ( * )
The non-equilibrium force can be attractive and repulsive. ( * )
Unlike in thermal equilibrium, there are points of stable levitation. ( * )
Forces are not equal and opposite, with points of equal force in the same direction! ( * )
![]() Example of non-equilibrium Casimir levitation:
Example of non-equilibrium Casimir levitation:

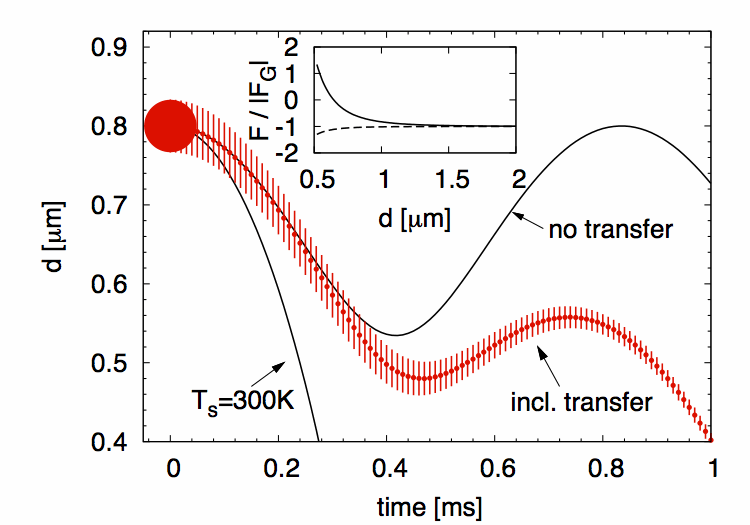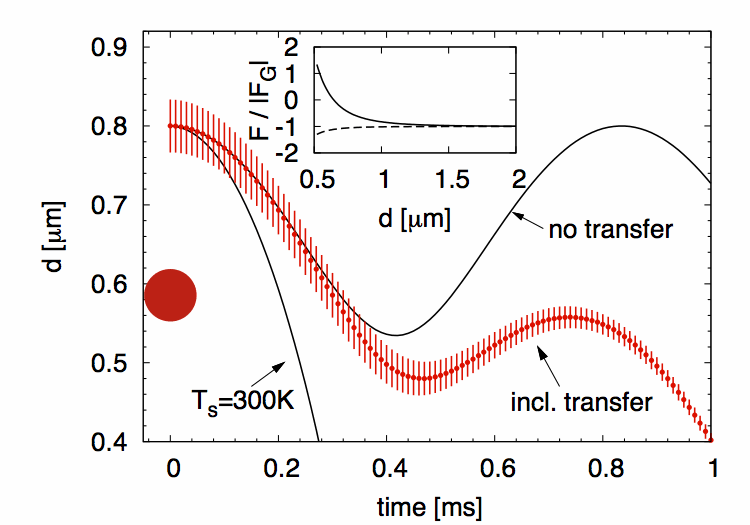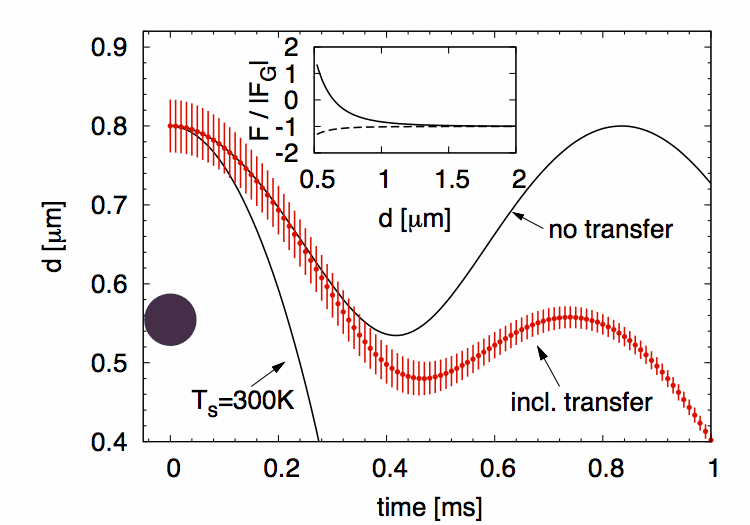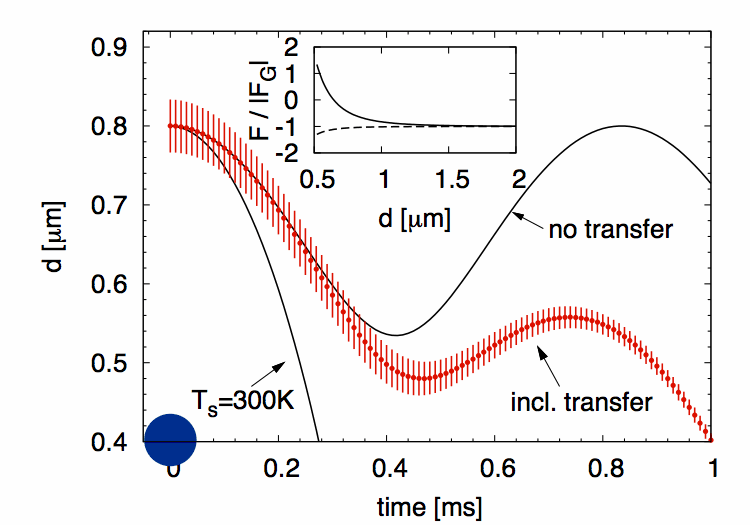
A hot microsphere can levitate on top of a cold plate, but falls if it cools down (due to heat transfer).