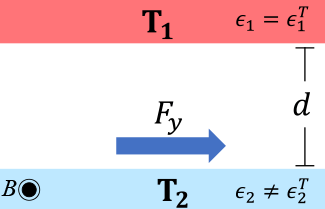
Power and efficiency in the near field

![]()
![]() For simplicity, the results will be expressed in terms of the Carnot efficiency:
For simplicity, the results will be expressed in terms of the Carnot efficiency:
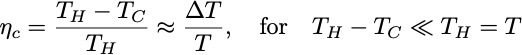
To extract power, the plate must move with some velocity v, leading to efficiency
A naive interpretation of the above formula is that the Carnot efficiency is reached at a velocity scale
![]() Onsager relations, however, imply that if heat exchange drives motion, motion must modify heat exchange according to
Onsager relations, however, imply that if heat exchange drives motion, motion must modify heat exchange according to
while there are also frictional forces (even with a vacuum gap) reducing the propulsive force to
![]() Focusing on a single material frequency
Focusing on a single material frequency ![]() , and expressing the degree of non-reciprocity in terms of a single dimensionless parameter
, and expressing the degree of non-reciprocity in terms of a single dimensionless parameter ![]() :
:

(C is a dimensionless function of hω/kT and reflectivities of the two bodies)
This leads to a final expression for the efficiency of

For maximum power
