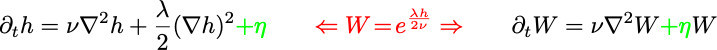
Cole-Hopf mapping
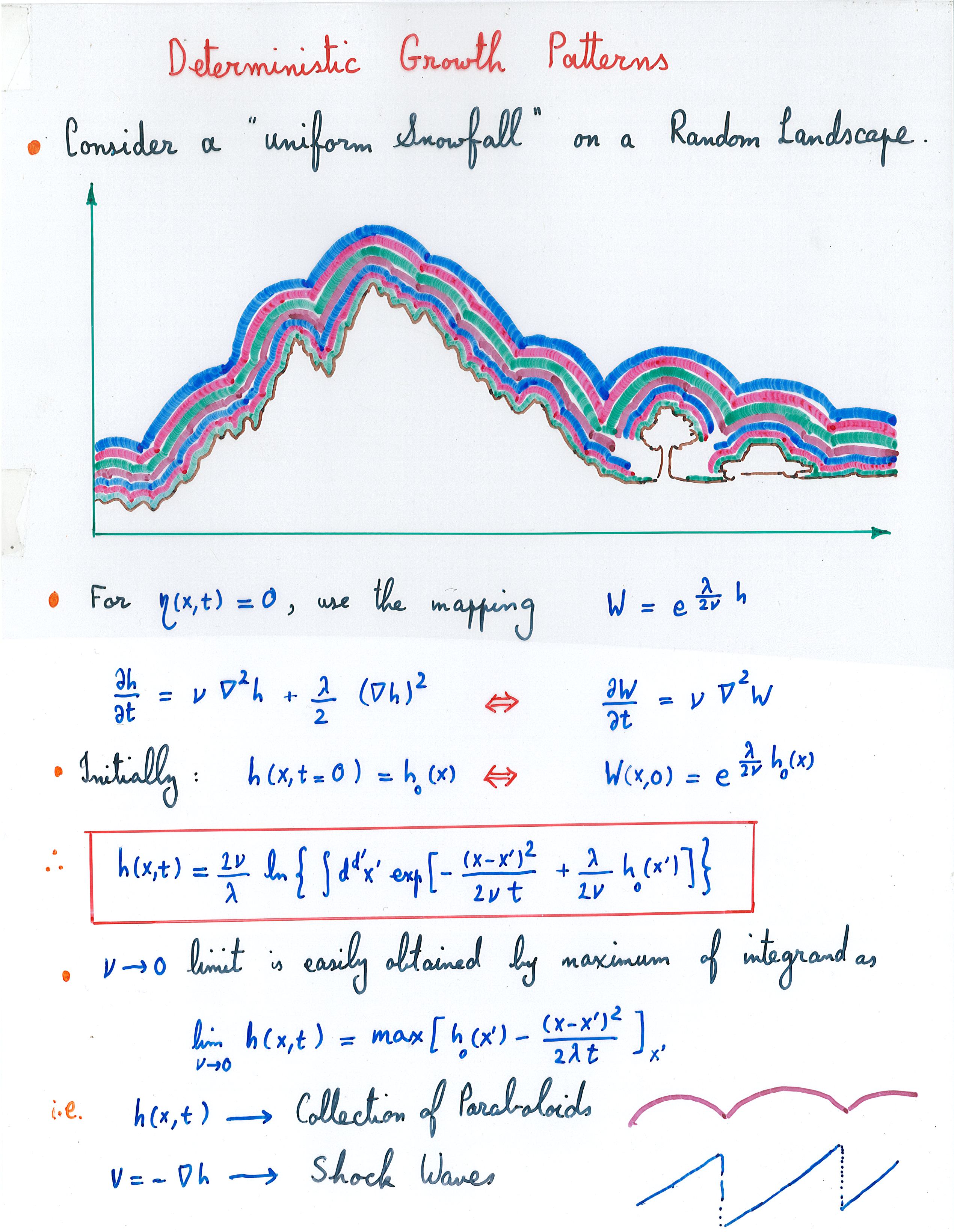
![]() Deterministic (noiseless) limit
Deterministic (noiseless) limit
![]() Can we construct such a mapping relating competitive growth equations to another model?
Can we construct such a mapping relating competitive growth equations to another model?
Linear (Eigen's) model of reproduction/mutation/diffusion of "quasi"-species:
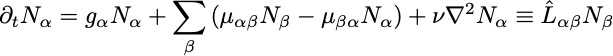
The linear deterministic equations are in principle exactly solvable:
However, the overall population at each location grows (decays) exponentially in time:
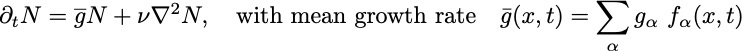
The species fractions at each location evolve as
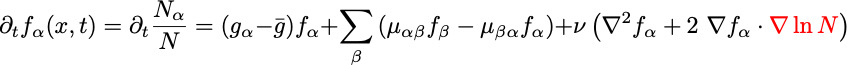
![]() A generalized Cole-Hopf transformation maps this linear problem to a variant of the range expansion model:
A generalized Cole-Hopf transformation maps this linear problem to a variant of the range expansion model:
=_2_nu_ove.jpeg)
This rough front is now coupled to species fractions according to
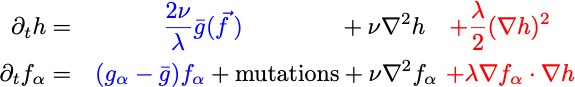
For two species, and no mutations, the corresponding limit of the general coupled equations takes the form:

Starting with an initial seeding of species on a rough surface, this deterministic variant of range expansion
can be solved exactly after a Cole-Hopf mapping to a corresponding linear "Eigen" model.

where =_h(x,.jpeg)
The coarsening pattern at longer times can be obtained by a saddle point approximation as
This describes a "circular arcs" advancing with velocity
The Cole-Hopf maps to exactly the point when the "circular arc" and "bare Fisher" velocities are identical!
A non-flat initial profile grows into a series of coarsening paraboloids:
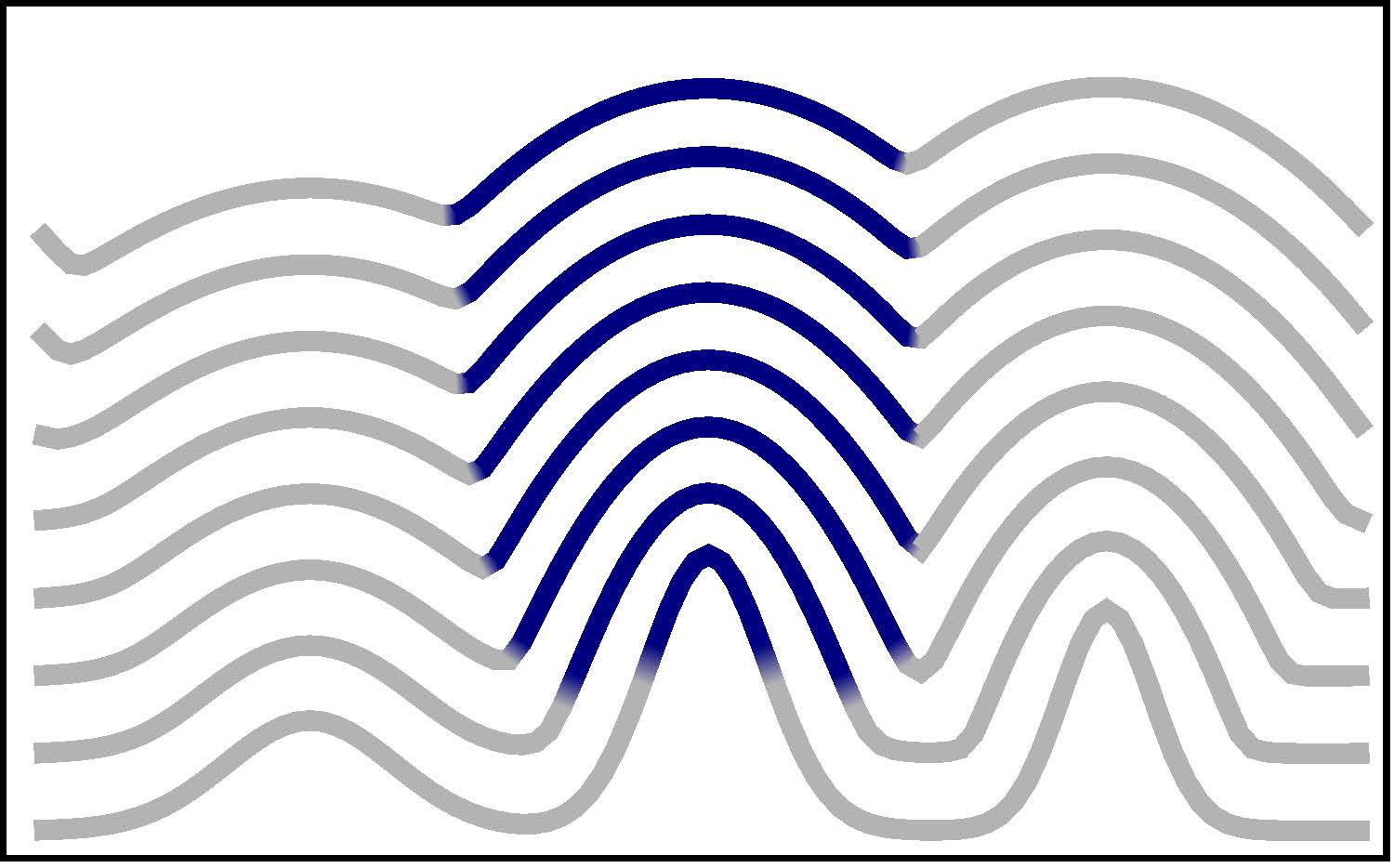
Each paraboloid is dominated by a single species located at an initial peak.
In the above picture, the blue species is less fit than the gray, and would have gone extinct on a flat front,
the advantage of initial location allows it to carve out its own geographic niche.
In this system the advantage of height h is equivalent to a exponentially larger seed population.
"Bacterial range expansions on a growing front: Roughness, Fixation, and Directed Percolation,"