Specialization during Range Expansion
![]() Novel traits evolve to take better advantage of resources, with concomitant changes in fitness
Novel traits evolve to take better advantage of resources, with concomitant changes in fitness
Assume that development of some traits can be indicated by parameter m=b-h ,
such that m=0 corresponds to the ancester (generalist),
while m>0 corresponds to one trait (e.g. height h>b), m<0 to another (e.g. breadth b>h)
Let us assume for simplicity that the dependence of fitness on m is expressed as the polynomial
![]()
The fitter parent in the stepping stone model wins the competition to reproduce
The child inherits traits from the parent, but also acquires mutational changes
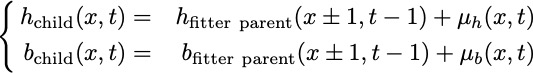
Starting with generalists ( m=0 ) at t=0 , specialists emerge in time through accumulating beneficial mutations:
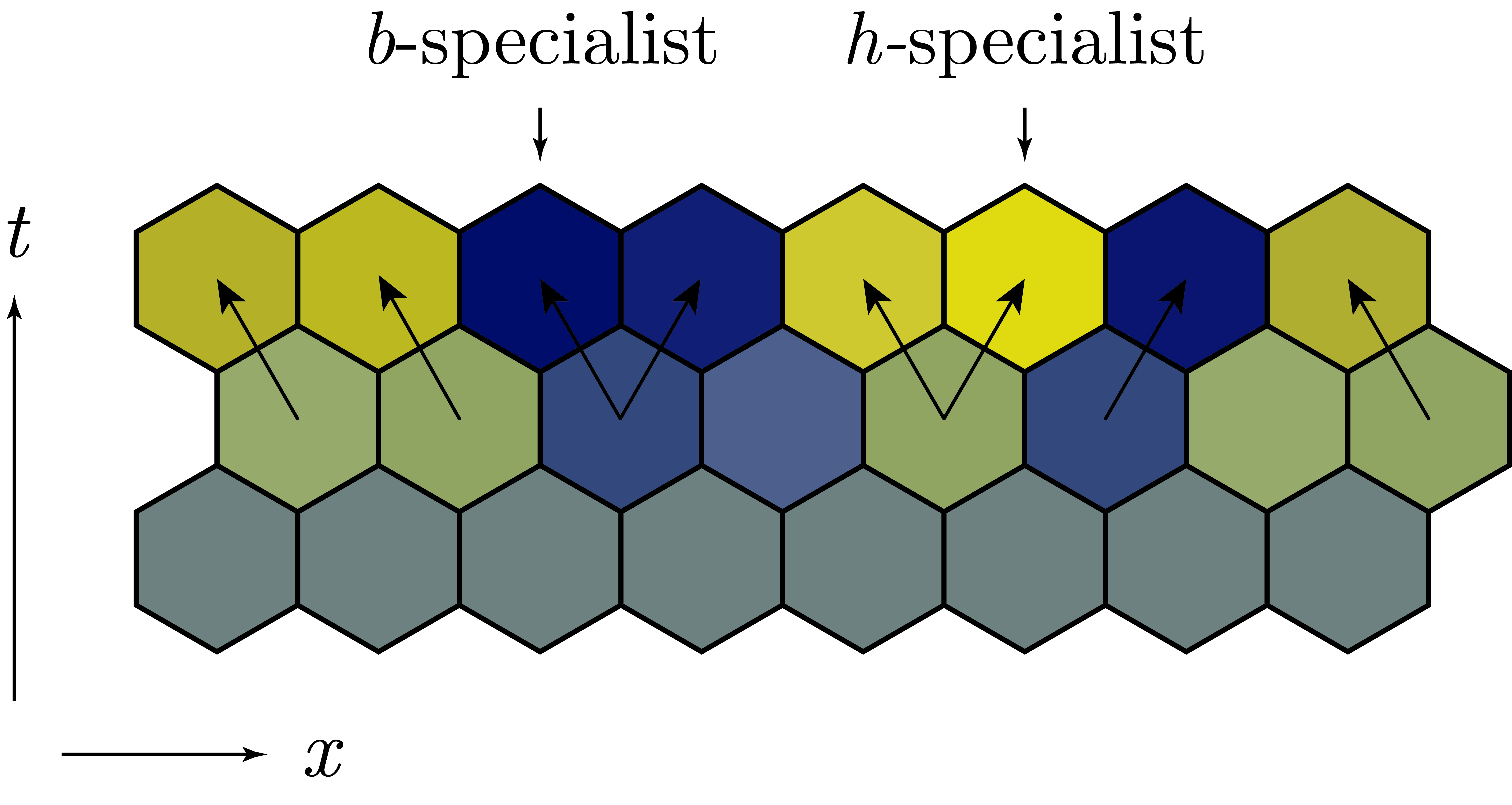
![]() Distinct growth patterns for different fitness function:
Distinct growth patterns for different fitness function:
1. Generalists fitness advantage (global)
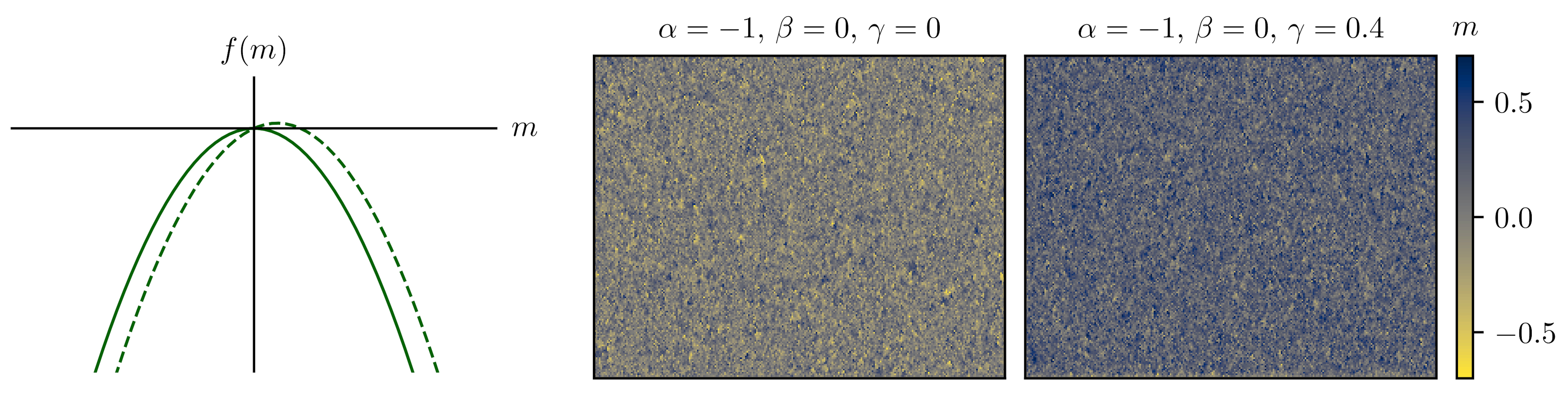
2. Specialists fitness advantage (unbounded)
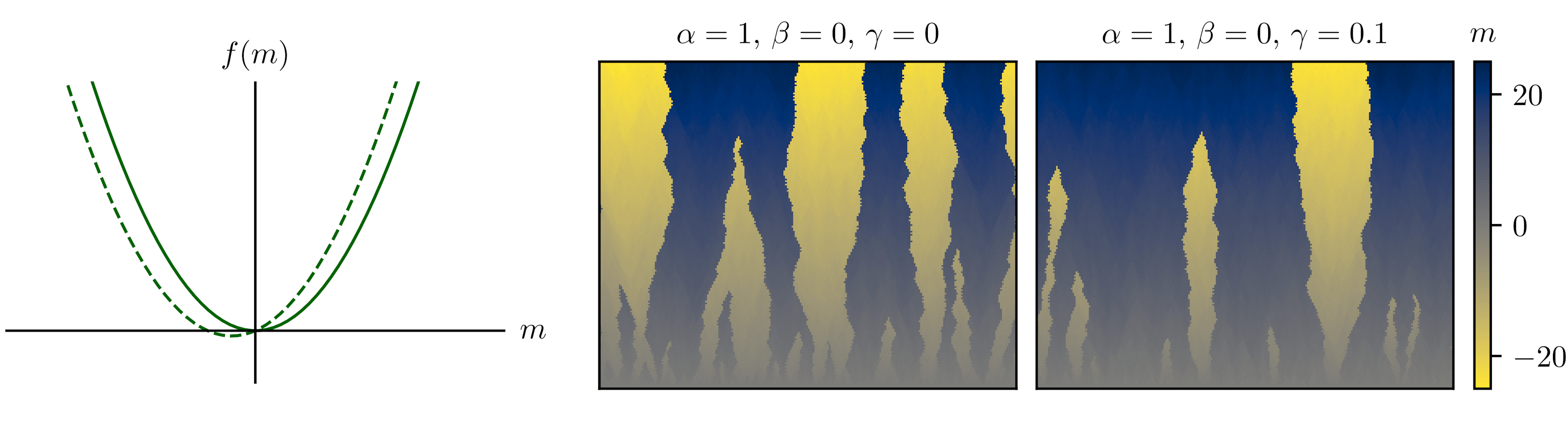
3. Specialists local fitness disadvantage, but global advantage
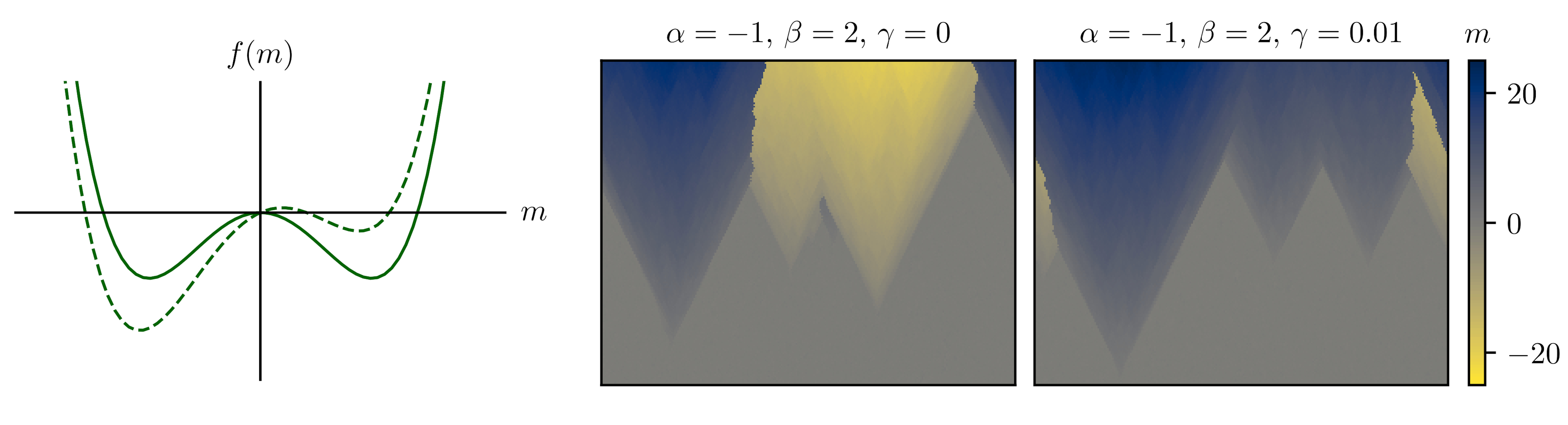
4. Specialists fitness advantage (bounded)

![]() Distinct universality classes for bounded and unbounded fitness gain:
Distinct universality classes for bounded and unbounded fitness gain:
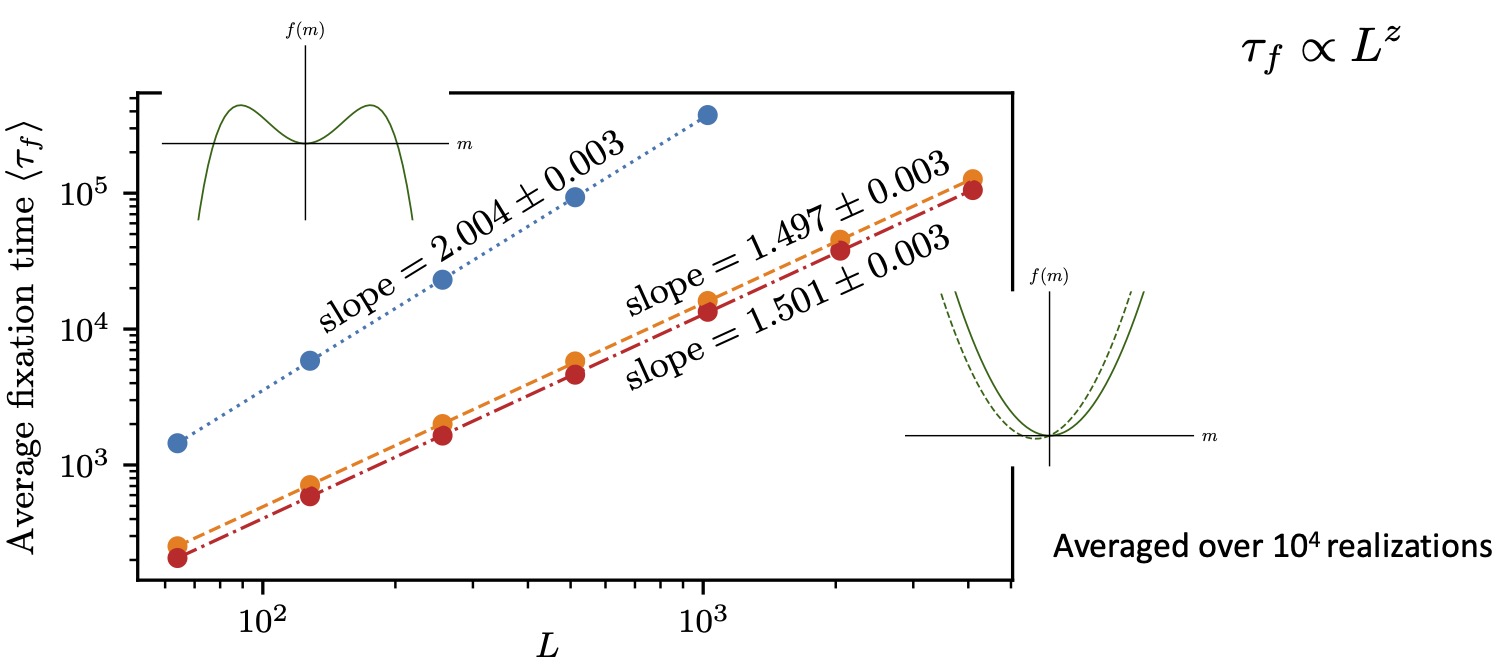
"Specializaton at an expanding population,"
Lauren H, Li and M. Kardar, Phys. Rev. E 108, L032402 (2023) (off-line)