Parallel Computing for Art

6.338J Project proposal

Ken Takusagawa

I have two projects listed here. The reason for this is that I am not sure yet how difficult each of the individual projects are. My very preliminary analysis suggests they each might be on the "easy" side.

First mini-project: Riemann zeta function audio

To create an (at-least) 74-minute recording of the Riemann zeta function evaluated along the critical line (Real = 0.5).

Details

• 74-minutes is the amount that can be burned onto a standard CD.
• The real and imaginary parts will go into separate stereo audio channels.
• A preliminary calculation suggests that the function will need to be evaluated out to 1-2 Million zeroes.
• This is an order of magnitude further than the largest other calculation I could find on the web, which went out to 100,000 zeroes. Furthermore, I will not only be calculating the zeroes, but the values of the function in between.
• A similar audio exploration of the zeta function was calculated by Robert Munafo, who produced an (only) 30 second MP3.
• Robert Munafo notes that the fundamental pitch of the zeta function increases logarithmically. Therefore, I plan to scale the time axis so that the fundamental pitch remains constant. This way, the pitch will not rise until it drifts out of the range of human hearing.
• One interesting parallel issue which I expect to face is load-balancing. I expect that some portions (probably larger imaginary component) of the zeta function will be more difficult to calculate than others.

Finally, although I lack the mathematical training to analyze the Riemann zeta function, the sound file (as a simple tabulation of values) may become a useful research tool to the mathematical community, for the purpose of forming and investigating conjectures.

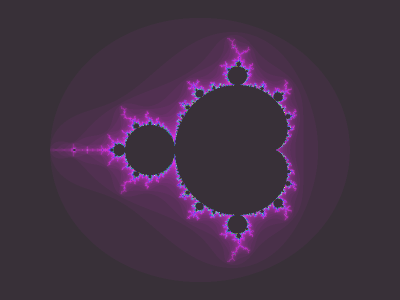
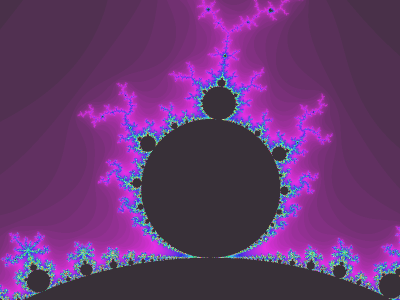
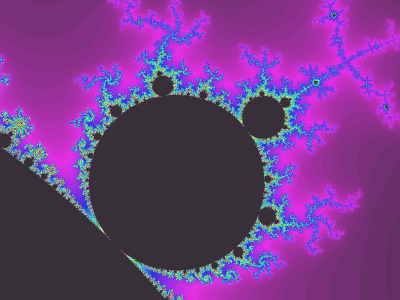
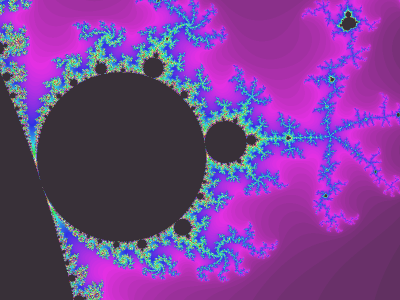
Second mini-project: Mandelbrot fractal

To create a very long and narrow (~1000x10000000) image of the crevice of a cardoid of the Mandelbrot set.

The buds which come off the cardoid of the Mandelbrot set exhibit pseudo self-similarity. That is, as one explores further and further into the crevice, the shape of the buds is almost the same: however, the "ornamentation" around the buds becomes increasingly intricate.

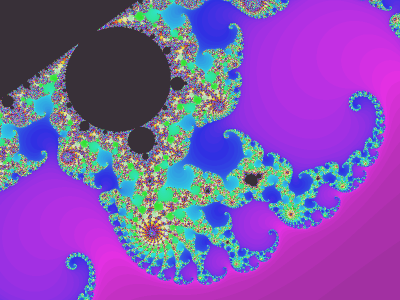
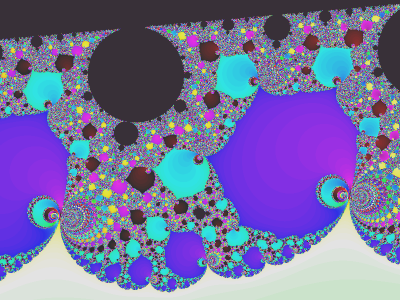
Because of self-similarity, once can imagine a warped infinite strip: The bottom edge of the strip runs along the edge of the cardoid. The top edge of the strip runs along the top of the buds. The strip is continuously scaled so that the buds remain the same size as one proceeds along the strip.

This warped strip can then be un-warped to produce a rectangular strip.

Details

• I conceived of this project about six years ago when I found a paper describing the formula for the cardoid, and the rate of self-similarity (i.e., at what rate the buds grow smaller). However, at the time, I lacked the resources necessary to carry out the computation.
• I actually won't be calculating the main cardoid of the Mandelbrot set. Instead I will be calculating a smaller (period 4(?)) cardoid which has more interesting ornametation around it. The formula for this smaller cardoid is a sextic polynomial, which is efficiently solved by Newton's method.
• There are several interesting parallel computation issues in this project.
  1. Load balancing: Different portions of the Mandelbrot set take considerably longer (by a factor of up to a billion) to calculate
  2. Can self-similarity be exploited to speed up the computation?
  3. Can parallel prefix be exploited to calculate the recurrence z := z² +c ?