Multiscale Modeling of Materials
| Personnel: | E.C. Allen, M. Bathe, F. Bernardin, S. Curgul, A.E.Ismail, K. Beers, A.J. Grodzinsky, Geo. Stephanopoulos, B. Tidor |
| Sponsorship: | DOE CSGF, NSF, DOD NDSEG (sponsor contact information) |
|
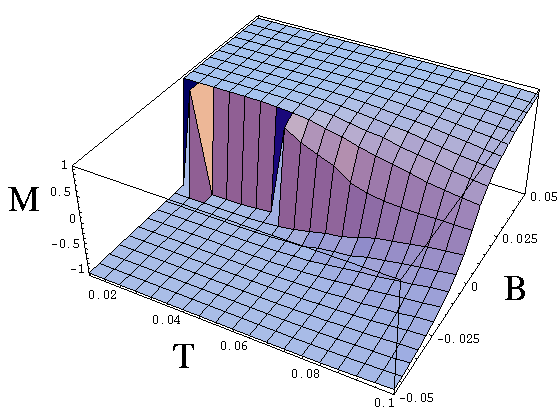
Figure: Phase diagram plotting average magnetization M vs temperature T and external field strength B for a 64364 ferromagnetic Ising lattice, computed using an (8,8)-model via WAMC. The general features correspond to those that would be produced with standard MMC, but require less than 3% of the computational time. |
The need for novel approaches to model polymers remains unabated even with the enormous increases in computational power of the last few years. While many multiscale approaches have been designed to study polymers, most of these are designed with very specific length or time scales in mind. Thus, interesting physical phenomena that occur in so-called "mesoscales" between the microscopic and macroscopic scales generally studied cannot be elucidated by such means. We have explored the development of multiscale modeling approaches that are either (a) algorithmically general or (b) chemically specific.
In the algorithm area, we previously employed the wavelet-accelerated Monte Carlo (WAMC) algorithm as a means of coarse-graining lattice systems [J. Chem. Phys. 118, 4414, and 4424, (2003)] and have now extended it to topologically coarse-grained systems such as polymers [J. Chem. Phys., 122, 234901 and 234902, (2005)]. The principal advantages of the WAMC methodology are flexibility and computational efficiency. The inherent flexibility of the model allows us to study polymer chains at any desired degree of resolution. Furthermore, the existence of scaling laws, which are readily obtained through simulations at different levels of resolution and for chains of different lengths, can be used to "map" results to resolutions which have not been directly simulated. Impressive gains can also be made in the efficiency of simulations: the hierarchical nature of our algorithm means that chain lengths that are normally impossible to simulate atomistically are readily handled via the WAMC algorithm. This is possible because, for the WAMC algorithm, both the time required to generate a new configuration and the number of configurations needed between uncorrelated states depends only on the number of coarse-grained variables being simulated at any one time.
To study aggrecan, a primary component of articular cartilage, we have employed a chemically specific approach to coarse-graining. The goal of this research is to employ molecular based modeling and simulation techniques to gain insight into the relationship between the chemical composition of aggrecan and its macroscopic structural and mechanical properties. Use of traditional, explicit atom models to achieve this goal is precluded by the high molecular weight. Here, we have focused on the glycosaminoglycan constituents of aggrecan, which constitute the largest fraction (approx. 90 percent) of its total molecular weight. In this approach, all-atom simulations of the solvated disaccharides constituting the glycosaminoglycans of interest demonstrate that the glycosidic torsion angles are the primary determinants of their conformational flexibility. Accordingly, we fix all other "hard" internal degrees of freedom in the all-atom description, resulting in a coarse-grained model in which the biopolymer conformation is determined solely by the set of glycosidic torsion angles. Glycosidic torsional potentials of mean force are computed from the torsion angle distribution functions obtained in the disaccharide simulations using Boltzmann-inversion. The mean center of charge of each monosaccharide is used in conjunction with Debye-Hueckel theory to model electrolyte solution-mediated non-bonded electrostatic interactions. These modeling assumptions enable the simulation of more than 1000 sugar monomers in an isolated chain and 30,000 monosaccharides in bulk, vastly greater system sizes than would be attainable with an all-atom representation (Figure 3). Results demonstrate that the developed coarse-grained model is capable of quantitatively predicting the dependence of persistence length on ionic strength, as well as the titration behavior and osmotic pressure of glycosaminoglycans [Biophys. J. 88, 3870 (2005)]. The model achieves a computational speed-up of over 100x with respect to all-atom simulations for single chains and enables the simulation of bulk systems of GAGs that are inaccessible using all-atom models.
Lastly, we have also taken an inverse approach to modeling systems in which information at one length scale (e.g from experiments or previous simulations) can be used to constrain the simulation on a different length scale. For this purpose, we can construct simulations in the SemiGrand canonical ensemble and perform Monte Carlo simulations (SGMC). This inverse approach was first used to include scattering information as a constraint on structure and produce an intermolecular potential for simple atomic [Phys. Rev. E 62, 021111, (2001)] and protein [J. Comput. Chem., 24, 876 (2003)] models. It was subsequently used to simulate polymer configuration evolution during stress-induced orientation relaxation on very long (by molecular terms), 1-10 sec time scales [Macromolecules 35, 6032 (2002)]. Currently, we are using the second moments of orientation distributions determined from birefringence measurements of dense melts to constrain the phase space by a single, global parameter. By constructing a reversible path in this global orientation parameter, we can determine the free energy difference between the isotropic and the oriented state. In one such application, oligomeric polyethylene is simulated subject to the observed second moment of orientation obtained from process simulations; the resulting ensembles can then be studied to understand how molecular level structure must change to conform to increasing levels of orientation.