Genetic Drift
Now we want to use the concept of a random walk to describe how a particular trait is passed through a population over time. The connection between the random walk and the evolution of a population is relatively straightforward. We can imagine that there is some probability that a particular trait will be passed on from one generation to the next. This is like the flip of the coin. So instead of starting with a large number of walkers with coins all starting from the same point you start with a large set of populations, all starting with the same fraction of individuals demonstrating a given trait. Instead of a coin flip and a step being our single time step, the birth of a new generation is our single time step.
Now for the sake of simplicity let’s say that the organisms we’re studying create new offspring asexually (no mating involved and the child of a particular member of the population is just a copy of that member) such that each member of the current population can create 0, 1 or more copies of itself to be placed in the next generation. Thus, there is some probability that there will be more of the trait being examined in the next generation (steps forward), less (steps back) or the same. This can be described with the same math as the poll of random walkers as they move back and forth. In an infinitely large population the distribution of the fraction of the population with the given trait will act exactly like the distribution of the position of the random walkers we described on the previous page.
Below on the left of the schematic, is a visualization of few generations of the type of evolution we’ve been discussing, but in a smaller population size of 8. Here green dots represent members of the population with the given trait and blue represent members without the given trait. An arrow denotes a member of the original population creating a copy of itself in the new generation.
In the context of evolution a population reaching the point where all the members have a given trait is known as fixation of that trait, while the population reaching a point where all the members do not have the given trait is known as loss of that trait. Though the math is more complicated than we’d like to go into here, the formalism of a random walk with adsorbing boundaries can be used to predict the average amount of time it takes for a population with an initial fraction exhibiting a trait to reach fixation or loss. We can also analyze the probability of fixation or loss occurring given the initial fraction of the population with the given trait. If the initial fraction of the population with a trait is high the probability of fixation of that trait is high and if the fraction is low the probability of loss is high. This is analogous to starting all of our walkers close to one or the other ice cream shop. Since the shop is not far from the initial position, a large number of the walkers hit it and stay there before they have the chance to explore much of the rest of the path.
For more information about genetic drift and some simulations see http://www.biology.arizona.edu/evolution/act/drift/dna_phenotype.html where this is explained using an evolving population of colored worms.
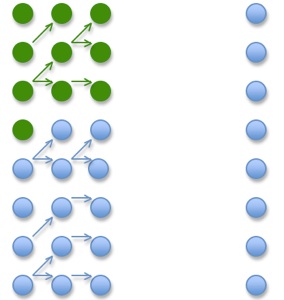
You may notice an interesting possibility depicted on the right side of the schematic. What if all the members of the new generation are of the same type? Doesn’t this change the dynamics of the evolution of the population? The answer to this is yes. With a fixed, finite population size, at some point the population could become completely made up of members with or without the trait we are tracking. Then it doesn’t matter how many copies each member creates to put in the next generation, the fraction of the population with/without that trait will remain at 1.
Never fear, this can still be described by a random walk, we just have to alter the setup a little bit. We need to describe it using a random walk with adsorbing boundaries. This sounds scary, but it’s very simple. Imagine instead of our random walkers from the previous page having an infinite line to step on, they have a line of a fixed number of spaces with a ice cream shop at either end of the line. When a walker reaches either ice cream shop instead of continuing to flip the coin and move, the walker will spend the coin on a cone and stay at the ice cream space to enjoy an afternoon snack.

Now the distribution will broaden with time as before, except once it broadens to the point where members start hitting the ice cream shops (or the particular population reaches a fraction of 0 or 1 of a given trait) walkers start piling up at the ice cream shops (or populations stop evolving and remain at a given fraction.) This evolution is shown in the animation below.