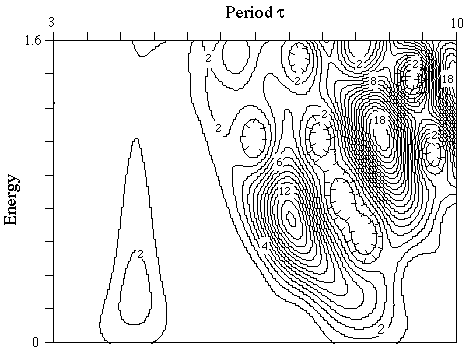
The classical periodic orbits are buried in the quantum mechanics in many ways, hence there are several ways to motivate and derive the quantal E-Tau plot. One way to do it is to send a Gaussian wavepacket (coherent state) along a trajectory. You time-evolve it quantum mechanically for the duration of one period tau, and then you calculate its overlap with the original packet. After summing over all possible trajectories (initial "locations" and "momenta" of the coherent states), you take the magnitude. Plotting this quantity vs. energy and period yields the quantal E-Tau plot.
This graph, instead of yielding a set of lines describing the families of periodic orbits as is the case with the classical plot, gives a contour graph or a 3-dimensional surface plot. In the limit as Planck's constant, h, goes to zero, this contour plot yields the classical plot (being non-zero only on the classical periodic orbits). However, for non-zero h, one sees interference effects between the "orbits." Below is a sample quantal E-Tau plot (this one is for the Nelson Hamiltonian):
