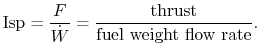Thermodynamics and Propulsion

14.1 Thrust and Specific Impulse for Rockets

Previously we used the steady flow energy equation to relate the exhaust velocity of a rocket motor, Figure 14.1, to the conditions in the combustion chamber and the exit pressure.

Figure 14.1: Schematic of rocket nozzle and combustion chamber

The steady flow energy equation

$$q_{\textrm{1-2}} - W_{\textrm{s,1-2}} = h_{T2} - h_{T1},$$

then with no heat transfer or shaft work,

$$h_{T2} = h_{T1} \quad \textrm{or} \quad h_{Tc} = h_{Te}$$

which can be written as

$$c_p T_c + \frac{u_c^2}{2} = c_p T_e + \frac{u_e^2}{2},$$

and manipulated to obtain

$$u_e = \sqrt{2c_p T_c\left[1-\left(\frac{p_e}{p_c}\right)^{\frac{\gamma-1}{\gamma}}\right]},$$

using

$$\frac{T_e}{T_c} = \left(\frac{P_e}{P_c}\right)^{\frac{\gamma-1}{\gamma}}.$$

Then considering the relationship we derived for thrust,

$$F = \dot{m} u_e + A_e (p_e - p_0),$$

and assuming $p_e = p_0 = 0$ , then

$$u_e = \sqrt{2c_pT_c},$$

$$F = \dot{m} u_e,$$

and

$$\textrm{Isp} = \frac{F}{\dot{W}}=\frac{\textrm{thrust}}{\textrm{fuel weight flow rate}}.$$

Rearranging,

$$\dot{m}u_e = \dot{W} \textrm{Isp}.$$

Thus the specific impulse can be directly related to the exhaust velocity leaving the rocket,

$$\textrm{Isp} = \frac{u_e}{g}.$$