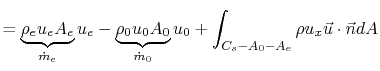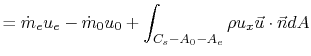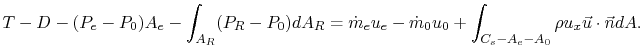Thermodynamics and Propulsion

10.3 Application of the Momentum Equation to an Aircraft Engine

Consider the jet engine shown in Figure 10.4.

Figure 10.4: Control volume for application of momentum theorem to an aircraft engine

$$\int_s \rho u_x(\vec{u}\cdot\vec{n})dA = T-D + \sum\textrm{Pressure Forces}$$

$$\int_s \rho u_x(\vec{u}\cdot\vec{n})dA = T-D+\left[-P_e A_e + P_0 A_e - \int_{A_R} (P_R-P_0)dA_R\right]$$

$$\int_s \rho u_x(\vec{u}\cdot\vec{n})dA = \underbrace{\rho_e u_e A_e}_{\dot{m}_e} u_e-\underbrace{\rho_0 u_0 A_0}_{\dot{m}_0} u_0 +\int_{C_s-A_0-A_e}\rho u_x\vec{u}\cdot\vec{n}dA$$

$$=\dot{m}_e u_e -\dot{m}_0 u_0 + \int_{C_s-A_0-A_e}\rho u_x \vec{u}\cdot\vec{n}dA$$

So we have:

$$T-D-(P_e-P_0)A_e-\int_{A_R}(P_R-P_0)dA_R = \dot{m}_e u_e-\dot{m}_0 u_0+\int_{C_s-A_e-A_0}\rho u_x\vec{u}\cdot\vec{n}dA.$$

Everything that relates to flow through the engine is conventionally called thrust. Everything that relates to the flow on the outside of the engine is conventionally call drag. Therefore, gathering only those terms that relate to the fluid that passes through the engine, we have:

$$T = \dot{m}_eu_e - \dot{m}_0 u_0 + (P_e-P_0)A_e.$$

The thrust is largely composed of the net change in momentum of the air entering and leaving the engine, with a typically small adjustment for the differences in pressure between the inlet and the exit. We could have arrived at the same equation by considering only the streamtube that passes through the engine as shown in Figure 10.5. Note that the static pressure along the curved control surfaces is different from ambient pressure due to streamline curvature.

Figure 10.5: Alternate control volume for application of momentum theorem to an aircraft engine