 |
 |
| TOILTETS | ||
| QUESTIONS OR COMMENTS | ||
 |
AUTHOR: | Lee Knight |
| E-MAIL: | pacosato@MIT.EDU | |
| COURSE: | 2A | |
| CLASS/YEAR: | 3 | |
MAIN FUNCTIONAL REQUIREMENT: Remove human waste
DESIGN PARAMETER: A toilet
GEOMETRY/STRUCTURE AND PARTS:
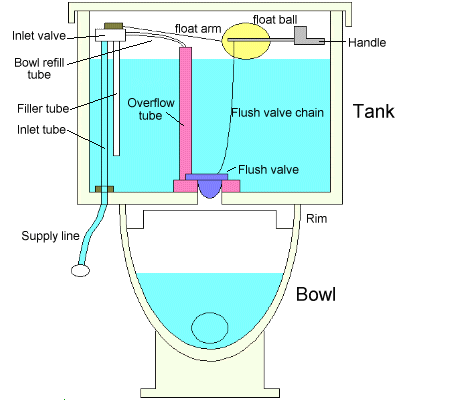 |
| Components of A Common Toilet |
EXPLANATION OF HOW IT WORKS/ IS USED:
To flush the toilet:
- When the handle is depressed, the flush valve chain opens the flush valve long enough for all the water in the tank (approximately 2 gallons) to rush into the toilet bowl.
- All the water is then siphoned through a tube connected to the sewer and out of the bowl (the concept of siphoning will be explained later). In flushing the toilet, the float ball drops due to the lack of water in the tank, opening the inlet valve to let more water into the tank. The flush valve closes after all the water has been emptied from the tank and the presence of the new water helps keep the valve closed.
- The water level increases (from the supply line, through the inlet tube and valve, passing through the bowl refill tube) until the float ball is floating on the water. When the water reaches a certain level, it causes the inlet valve to close and stops the tank from filling further.
- If the inlet valve did not shut off, and the water kept flowing into the tank, the overflow tube would prevent it from doing so.
How a siphon works:
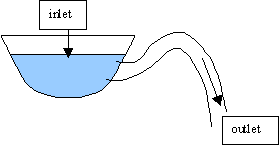 |
| Example of A Simple Siphon |
- Siphoning is used in transporting a fluid from a reservoir of higher elevation to one of lower elevation. Bernoulli’s equation (shown below) can help explain this type of fluid transport.
- The siphon for a toilet is an upside down "U" shaped tube that connects the bowl (higher reservoir) to the sewer/cespool (lower reservoir).
- If fluid (anything under approximately 2 gallons) is added to the toilet bowl itself, notice that the water level does not change. The extra fluid will cause the water level in the bowl to rise slightly for a moment, but then that extra water overflows into the siphon tube, passes through the tube, and out of the system (but this does not cause the toilet to flush).
- When the toilet is flushed, 2 gallons of water rush quickly through the bowl, causing the siphon tube to fill up completely, resulting in a change in pressure (lower pressure inside the tube ahead of the flowing water, and higher pressure in the water (at the inlet of the siphon) in the bowl. Once all the water has passed through the tube, air enters and interrupts the siphoning process (causing the gurgle you hear after you flush). Then water in the bowl is replenished when the inlet valve in the tank allows more water to come in through the supply line.
DOMINANT PHYSICS:
See also siphoning above
| Units | |||
| Variable | Variable Description: | Metric | English |
| P in | Pressure at inlet | Pa | psi |
| P out | Pressure at outlet | Pa | psi |
| V in | Velocity of fluid at inlet | m/s | ft/s |
| V out | Velocity of fluid at outlet | m/s | ft/s |
| Z in | Height of the inlet | m | ft |
| Z out | Height of the outlet | m | ft |
| p | Density of the fluid | kg/m3 | lbm/m3 |
| h loss | Head loss (losses due to friction) | m2/s2 | ft2/s2 |
Bernoulli’s Equation:
![]()
With regard to a toilet, we can assume that the density (p) of water is constant, gravity (g) is constant, h loss and v in are both negligible compared to the other terms, and p in is atmospheric pressure. I timed the toilet in my house and it took approximately 8 seconds. Since we know that it takes 8 seconds for 2 gallons of water to flow through the cross sectional area of the pipe, we can determine a rough estimate for the flow rate (Q). Knowing Q and assuming the cross sectional Area, we can estimate the average velocities at specific points.
Flow rate:
Q = A*v
Since the flow rate (Q) is constant throughout the system, according to
the law of conservation of mass (![]() ) and assuming that the density
(p) remains constant, we can state that:
) and assuming that the density
(p) remains constant, we can state that:
A*v in = A* v out
LIMITING PHYSICS:
Factors that may limit the performance of the toilet would include the cross sectional area of the bowl, the amount of water used to flush the toilet, and the time it takes for all the water to pass through. The mechanical efficiency is not that important in the case of the toilet.
Friction:
The friction between water and the siphon pipe is relatively low and can be determined using the Reynolds number.
![]()
Where v is the velocity of the fluid, d is the diameter of the pipe the fluid is flowing through, p is the density of the fluid (water=1000 kg/m^3), and m is the viscosity of the fluid (water=1x10-3 kg/m*s). When the Reynolds number is less than 2300 (as in a toilet), it is considered laminar flow and friction forces are assumed to be negligible.
PLOTS/GRAPHS/TABLES:
None Submitted
WHERE TO FIND TOILETS:
Hopefully you will allow yourself enough time to locate one. Suggestions would include the W.C., behind a tree, next to the food court in the mall, and in worst case scenario, you can always ask.
REFERENCES/MORE INFORMATION:
http://howthingswork.virginia.edu