11.0
Introduction
One way to decide whether a system is electroquasistatic or magnetoquasistatic is to consider the relative magnitudes of the electric and magnetic energy storages. The subject of this chapter therefore makes a natural transition from the quasistatic laws to the complete set of electrodynamic laws. In the order introduced in Chaps. 1 and 2, but now including polarization and magnetization,
1 For polarized and magnetized media at rest. these are Gauss' law [(6.2.1) and (6.2.3)]
Ampère's law (6.2.11),
Faraday's law (9.2.7),
and the magnetic flux continuity law (9.2.2).
Circuit theory describes the excitation of a two-terminal element in terms of the voltage v applied between the terminals and the current i into and out of the respective terminals. The power supplied through the terminal pair is vi. One objective in this chapter is to extend the concept of power flow in such a way that power is thought to flow throughout space, and is not associated only with current flow into and out of terminals. The basis for this extension is the laws of electrodynamics, (1)-(4).
Even if a system can be represented by a circuit, the need for the generalization of the circuit-theoretical power flow concept is apparent if we try to understand how electrical energy is transferred within, rather than between, circuit elements. The limitations of the circuit viewpoint would be crucial to testimony of an expert witness in litigation concerning the authority of the Federal Power Commission
2Now the Federal Energy Regulatory Commission. to regulate power flowing between states. If the view is taken that passage of current across a border is a prerequisite for power flow, either of the devices shown in Fig. 11.0.1 might be installed at the border to "launder" the power. In the first, the state line passes through the air gap between capacitor plates, while in the second, it separates the primary from the secondary in a transformer.
3To be practical, the capacitor would be constructed with an enormous number of interspersed plates, so that in order to keep the state line in the air gap, a gerrymandered border would be required. Contemplation of the construction of a practical transformer, as described in Sec. 9.7, reveals that the state line would be even more difficult to explain in the MQS case. In each case, the current never leaves the state where it is generated. Yet in the examples shown, power generated in one state can surely be consumed in another, and a meaningful discussion of how this takes place must be based on a broadened view of power flow.
From the circuit-theoretical viewpoint, energy storage and rate of energy dissipation are assigned to circuit elements as a whole. Power flowing through a terminal pair is expressed as the product of a potential difference v between the terminals and the current i in one terminal and out of the other. Thus, the terminal voltage v and current i do provide a meaningful description of power flow into a surface S that encloses the circuit shown in Fig. 11.0.2. The surface S does not pass "inside" one of the elements.
Power Flow in a Circuit
For the circuit of Fig. 11.0.2, Kirchhoff's laws combine with the terminal relations for the capacitor, inductor, and resistor to give
Motivated by the objective to obtain a statement involving vi, we multiply the first of these laws by the terminal voltage v. To eliminate the term viL on the right, we also multiply the second equation by iL. Thus, with the addition of the two relations, we obtain
Because L and C are assumed to be constant, we can use the
relation udu = d(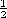
u2) to rewrite this expression as
where
With its origins solely in the circuit laws, (8) can be regarded as giving no more information than inherent in the original laws. However, it gives insights into the circuit dynamics that are harbingers of what can be expected from the more general statement to be derived in Sec. 11.1. These come from considering some extremes.
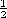Cv2 and the magnetic energy 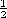LiL2
is constant.
Because the right-hand side is a perfect time derivative, the expression can be integrated to give
Regardless of the details of how the currents and voltage vary with time, the time integral of the power vi is solely a function of the initial and final total energies w. Thus, if w were zero to begin with and vi were positive, at some later time t, the total energy would be the positive value given by (10). To remove the total energy from the inductor and capacitor, vi must be reversed in sign until the integration has reduced w to zero. Because the process is reversible, we say that the energy w is stored in the capacitor and inductor.
In circuit theory terms, (8) is an example of an energy conservation theorem. According to this theorem, electrical energy is not conserved. Rather, of the electrical energy supplied to the circuit at the rate vi, part is stored in the capacitor and inductor and indeed conserved, and part is dissipated in the resistor. The energy supplied to the resistor is not conserved in electrical form. This energy is dissipated in heat and becomes a new kind of energy, thermal energy.
Just as the circuit laws can be combined to describe the flow of power between the circuit elements, so Maxwell's equations are the basis for a field-theoretical view of power flow. The reasoning that casts the circuit laws into a power flow statement parallels that used in the next section to obtain the more general field-theoretical law, so it is worthwhile to review how the circuit laws are combined to obtain a statement describing power flow.
Overview
The energy conservation theorem derived in the next two sections will also not be a conservation theorem in the sense that electrical energy is conserved. Rather, in addition to accounting for the storage of energy, it will include conversion of energy into other forms as well. Indeed, one of the main reasons for our interest in power flow is the insight it gives into other subsystems of the physical world [e.g. the thermodynamic, chemical, or mechanical subsystems]. This will be evident from the topics of subsequent sections.
The conservation of energy statement assumes as many special forms as there are different constitutive laws. This is one reason for pausing with Sec. 11.1 to summarize the integral and differential forms of the conservation law, regardless of the particular application. We shall reference these expressions throughout the chapter. The derivation of Poynting's theorem, in the first part of Sec. 11.2, is motivated by the form of the general conservation theorem. As subsequent sections evolve, we shall also make continued reference to this law in its general form.
By specializing the materials to Ohmic conductors with linear polarization and magnetization constitutive laws, it is possible to make a clear identification of the origins of electrical energy storage and dissipation in media. Such systems are considered in Sec. 11.3, where the flow of power from source to "sinks" of thermal dissipation is illustrated. Processes of energy storage and dissipation are developed in greater depth in Secs. 11.4 and 11.5.
Through Sec. 11.5, the assumption is that materials are at rest. In Secs. 11.6 and 11.7, the power input is studied in the presence of motion of materials. These sections illustrate how the energy conservation law is used to determine electric and magnetic forces on macroscopic media. The discussion in these sections is confined to a determination of total forces. Consistent with the field theory point of view is the concept of a distributed force per unit volume, a force density. Rigorous derivations of macroscopic force densities are based on energy arguments paralleling those of Secs. 11.6 and 11.7.
In Sec. 11.8, we shall look at microscopic models of force density distributions that provide a picture of the origin of these distributions. Finally, Sec. 11.9 is an introduction to the macroscopic force densities needed to put electromechanical coupling on a continuum basis.