4.12 Imposing fees at a congested facility It is often
necessary to impose
congestion tolls to assure that expensive transportation facilities
(tunnels, bridges,
terminal space at bus terminals, etc.) are used efficiently.
Consider a city-owned airport
and assume that this airport has a runway that is used only for
landings during the peak
traffic hours. Under peak conditions, the arrivals of airplanes at
the vicinity of the
airport are assumed to be Poisson with a rate 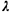 = 55 aircraft/hour. Of
these airplanes, 40 on
the average are commercial jets and 15 are small private airplanes
("general
aviation"). The pdf for the duration of the service time to a
random aircraft landing
on the runway is given by the uniform pdf of Figure P4.12(a). = 55 aircraft/hour. Of
these airplanes, 40 on
the average are commercial jets and 15 are small private airplanes
("general
aviation"). The pdf for the duration of the service time to a
random aircraft landing
on the runway is given by the uniform pdf of Figure P4.12(a).
a. Peak-hour conditions occur during 1,000 hours/year and the
average cost of 1
minute's waiting (i.e., "going into a holding pattern"
near the airport) is $12
for commercial jets (this accounts primarily for the extra fuel
spent, the extra flight
crew time and extra aircraft maintenance costs). Estimate the
yearly costs to the airlines
of peak-hour delays at this runway.
b. To alleviate peak-hour congestion, the airport's managers
are considering an
across-the-board increase in the fees that aircraft pay for using
the runway ("landing
fees"). While airline demand is insensitive to moderate
increases in landing fees,
general aviation demand is expected to drop drastically as these
fees increase (since
there are some good small airports near the city in question which
can be used by small
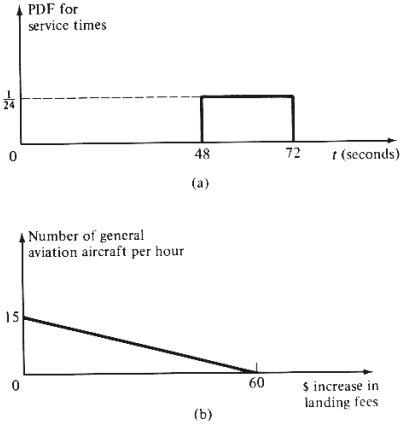
aircraft). In fact, a study of the general aviation runway users
revealed that the
relationship between demand per hour by general aviation and
increase in landing fee is as
shown in Figure P4.12(b). What is the most desirable amount of
increase in landing fees
from the point of view of the airlines? (Remember that the
airlines will also be paying
the higher fees.)
|