

|
|
| Thermodynamics and Propulsion | |
The second type of heat transfer to be examined is convection, where
a key problem is determining the boundary conditions at a surface
exposed to a flowing fluid. An example is the wall temperature in a
turbine blade because turbine temperatures are critical for creep
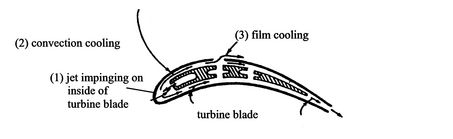
(and thus blade) life. A view of the problem is given in
Figure 17.1, which shows a
cross-sectional view of a turbine blade. There are three different
types of cooling indicated, all meant to ensure that the metal is
kept at a temperature much lower than that of the combustor exit
flow in which the turbine blade operates. In this case, the turbine
wall temperature is not known and must be found as part of
the solution to the problem.
|
|
To find the turbine wall temperature, we need to analyze convective
heat transfer, which means we need to examine some features of the
fluid motion near a surface. The conditions near a surface are
illustrated schematically in Figure 17.2. In a
region of thickness ![]() , there is a thin ``film'' of slowly
moving fluid through which most of the temperature difference
occurs. Outside this layer,
, there is a thin ``film'' of slowly
moving fluid through which most of the temperature difference
occurs. Outside this layer, ![]() is roughly uniform (this defines
is roughly uniform (this defines
![]() ). The heat flux can thus be expressed as
). The heat flux can thus be expressed as
It cannot be emphasized enough that this is a very crude picture.
The general concept, however, is correct, in that close to the wall,
there is a thin layer in which heat is transferred basically by
conduction. Outside of this region is high mixing. The difficulty is
that the thickness of the layer is not a fluid property. It depends
on velocity (Reynolds number), structure of the wall surface,
pressure gradient and Mach number. Generally ![]() is not known
and needs to be found and it is customary to calculate the heat
transfer using
is not known
and needs to be found and it is customary to calculate the heat
transfer using
![]() . This quantity has the
symbol
. This quantity has the
symbol ![]() and is known as the convective heat transfer coefficient.
The units of
and is known as the convective heat transfer coefficient.
The units of ![]() are W/m2K. The convective heat
transfer coefficient is defined by
are W/m2K. The convective heat
transfer coefficient is defined by
Muddy Points
How do we know that ![]() is not a fluid property?
(MP 17.1)
is not a fluid property?
(MP 17.1)