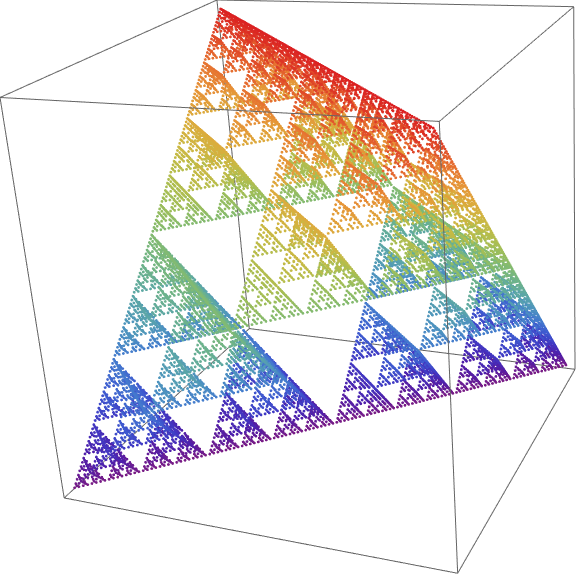
 The Sierpinksky tetrathedron
The Sierpinksky tetrathedron
Abstract
The multistochastic $ (n,k)$-Monge–Kantorovich problem on a product space $\prod_{i=1}^n X_i$ is an extension of the classical Monge–Kantorovich problem. This problem is considered on the space of measures with fixed projections onto $X_{i_1} \times \ldots \times X_{i_k}$ for all $k$-tuples ${i_1, \ldots, i_k} \subset {1, \ldots, n}$ for a given $1 \le k < n$. In our paper we study well-posedness of the primal and the corresponding dual problem. Our central result describes a solution $\pi$ to the following important model case $n=3, k=2, X_i = [0,1]$, the cost function $c(x,y,z) = xyz$, and the corresponding two–dimensional projections are Lebesgue measures on $[0,1]^2$. We prove, in particular, that the mapping $(x,y) \to x \oplus y$, where $\oplus$ is the bitwise addition (xor- or Nim-addition) on $[0,1] \cong \mathbb{Z}_2^{\infty}$, is the corresponding optimal transportation. In particular, the support of $\pi$ is the Sierpi'nski tetrahedron. In addition, we describe a solution to the corresponding dual problem.