| (118) |
The governing equations for diffusion mass transfer are [32]
| (118) |
and
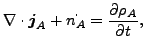 |
(119) |
where
| (120) |
and
| (121) |
In these equations
![]() is the mass flux of species A,
is the mass flux of species A,
![]() is the mass diffusivity,
is the mass diffusivity, ![]() is the mass fraction of species A and
is the mass fraction of species A and ![]() is the density of species A. Furthermore,
is the density of species A. Furthermore, ![]() is the rate of increase
of the mass of species A per unit volume of the mixture. Another way of
formulating this is:
is the rate of increase
of the mass of species A per unit volume of the mixture. Another way of
formulating this is:
| (122) |
and
 |
(123) |
where
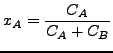 |
(124) |
and
| (125) |
Here,
![]() is the molar flux of species A,
is the molar flux of species A,
![]() is the mass diffusivity,
is the mass diffusivity, ![]() is the mole fraction of species A and
is the mole fraction of species A and ![]() is the molar concentration of species A. Furthermore,
is the molar concentration of species A. Furthermore, ![]() is the rate of increase
of the molar concentration of species A.
is the rate of increase
of the molar concentration of species A.
The resulting equation now reads
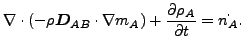 |
(126) |
or
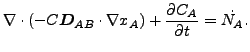 |
(127) |
If ![]() and
and ![]() are constant, these equations reduce to:
are constant, these equations reduce to:
 |
(128) |
or
 |
(129) |
Accordingly, by comparison with the heat equation, the correspondence in Table (16) arises.