Theory
I. Background
Among one of the most studied and marveled at biological materials is spider silk, which is known for being extremely tough and extensible, even surpassing steel and other industrial materials. These properties can be seen by sheer observation, judging from the fact that spiders use their silk to support their own weight, and even absorb kinetic energy to capture prey.
Scientists are currently exploring these mechanical properties and how they can be channeled in various ways, including tissue regeneration, medical sutures, parachutes, and other biomedical applications. Spider silk is an especially important biomaterial because of its degradability. The exploration of this property has caused silk biomaterials to be successfully employed in wound healing as well as tissue engineering.
The hierarchical structure of the silk is crucial in order for the silk to retain its mechanical properties. This structure consists of extremely organized, hydrogen-bonded beta-sheet nanocrystals arranged within a semi-amorphous protein matrix consisting of less organized protein structures.
Deformation of Spider Silk

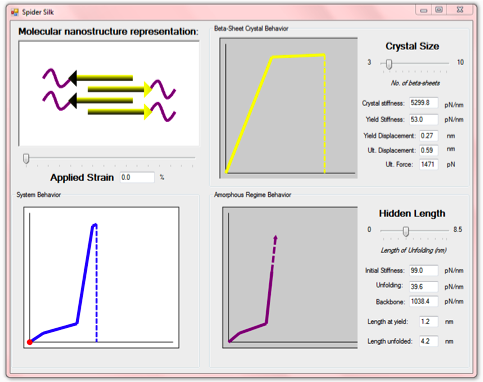
Simulation Instructions
To download this application, press on the link above.
Once launched, the application is dynamic and constantly running.
Once launched, this interactive application will react to user input via the mouse, you can either:
1. Adjust the represented silk “crystal size” from 3 nm to 10 nm, which changes
the mechanical response accordingly;
2. Adjust the represented silk “hidden length”, which corresponds to the
unfolding of amorphous (e.g. coiled and tangled) protein domains;
3. Apply strain to the silk nanostructure and see the deformation modes and
corresponding force levels.
In the molecular nanostructure representation (upper left) consists of four beta-sheet protein domains, as well as attached semi-amorphous region.
The upper right plot shows the bilinear spring behavior of the beta-sheet nanocrystals.
The lower right plot shows the trilinear behavior of the semi-amorphous region of the spider silk.
Finally, the lower left plot displays the behavior of the entire spider silk system, combining the behaviors of the two defining regions.
Download SpiderSilk.exe
Simulation Description
This simulation is meant to demonstrate a simple nonlinear spring representation of
spider silk. By changing the behavior of each of the characteristic regions (see
descriptions below) we can learn a lot about the forces applied on spider silk and
how they can cause the silk to deform. Four phases of deformation can be seen in
this demonstration.
Credits
Program Author(s):
Webpage Author(s):
Advisor:
Citations
[1] Andrea Nova, Sinan Keten, Nicola M. Pugno, Alberto Redaelli, Markus J. Buehler, 2010. Molecular and Nanostructural Mechanisms of Deformation, Strength and Toughness of Spider Silk Fibrils. Nano Letters 10 (7), 2626-2634.
[2] Keten, S. and M.J. Buehler, Nanostructure and molecular mechanics of dragline spider silk protein assemblies. Journal of the Royal Society Interface, 2010., in press, published online June 2, 2010, doi: 10.1098/rsif.2010.0149
[3] Sinan Keten, Markus Buehler, 2010. Atomistic Model of the Spider Silk Nanostructure. Applied Physics Letters 96.
[4] S. Keten, Z. Xu, B. Ihle, M. J. Buehler, "Nanoconfinement controls stiffness, strength and mechanical toughness of β-sheet crystals in silk", Nature Materials, 2010, 9, p. 359-367.





Copyright (c) 2010 Laboratory of Atomistic and Molecular Mechanics. All rights reserved.

III. Coarse-Grained Mesoscale Model - Nonlinear Springs in Series
The lab has developed ways to study spider silk and demonstrate how it actually deforms on a nanoscale level. Through various tests using differing beta-sheet nanocrystal sizes, the lab has determined that a “coarse-grained” model can be implemented to represent the deformation of the spider silk system, as opposed to a full atomistic representation (this is due to the fact that the results from each test still showed the characteristic stress-strain graphs).
Though this course-grained model does not take into account many variables in spider silk deformation, it is quite useful and relevant in this context, as it does explore the role of beta-sheet nanocrystal size (the key factor in deformation rate) on deformation. What we have
adapted is known as a “bottom-up” approach, as we are exploring silk on the fundamental level.
The downloadable application we created explains the theory behind our coarse-grained model. Our model represents the semi-amorphous and crystal regions of the spider silk as beads connected by nonlinear springs in a serial arrangement. This model reduces the tensile loading of the spider silk to nothing more than a force-displacement model between two springs, which can be translated into stress-strain values.
The displacement of the “springs” or “amorphous” regions depends on the stiffness of those regions. In nonlinear spring models however, the total stiffness changes as a function of strain; it is necessary to know the strain to know if that particular spring has yielded or not. It is reasonable to use a serial spring model in this instance because at the nanoscale the crystals are connected by repeating protein sequences.
The characteristics of the silk are due to the order of the amino acid residues in the protein sequence of silk: the poly-(Glycine-Alanine) and poly-Alanine domain makeup of the beta-sheet nanocrystals.
One of the current ways to analyze the properties of spider silk is by using a one-dimensional coarse-grained model, which essentially models the beta-sheet nanocrystal and semi-amorphous regions using beads connected by nonlinear springs.
Through a number of molecular dynamics simulations, it has been determined that the physical size of the beta-sheet nanocrystals affects the tensile strength of the silk as a whole. When the beta-sheet nanocrystals are smaller in size is when the spider silk is the toughest mechanically, highest in strength, and stiffest. The importance of the beta-sheet nanocrystals is highly evident when stretching of the silk occurs; they reinforce the partially extended macromolecular chains by forming interlocking regions that transfer the force load between the chains, which allows for greater extensibility of the amorphous region. The one-dimensional model that will be explained in greater detail reveals that the semi-amorphous region dominates deformation at small deformation levels (the region starts to unravel when the silk is stretched), but the beta-sheet nanocrystals dominate after the semi-amorphous region has essentially stretched to capacity. When an axial force is applied to spider silk, the silk experiences four distinct regimes that include a high tangent modulus, a softening effect, a stiffening effect, and then finally complete failure.
II. Four Deformation Stages
After extensive experiments of applying loads to spider silk researchers have observed that deformation in the silk experiences four distinct regimes, each with its own characteristic.
i. The first behavior noticed is that when the silk is first stretched, the silk experiences
a high tangent modulus. The occurring stretching of the silk causes the hydrogen
bonds in the semi-amorphous regions to rupture. As the helices and beta-turns in
the semi-amorphous domain of spider silk are quite rich in hydrogen bonds, the
initial regime culminates in an early yield point for the silk (at strain values of about
15%). This yielding causes a significant decrease in the tangent stiffness of the silk,
which then leads into the second regime of deformation.
ii. The second (plateau) regime is characterized by an actually observable softening of
the physical nanostructure of the spider silk; this physical behavior is a direct result
of the early yielding point inherent to the first regime. If we drill-down to a
nanoscale level, we can see that the protein chains in the semi-amorphous region
actually start to align with the direction the load was applied. This process is
gradual, due to the significant yet hidden length of the polypeptide chains; the
polypeptide length allows the silk to still experience low stress values despite
increases in strain values, hence why this regime is also known as the plateau
regime. Up to this point the behavior of the silk has almost been completely
dependent upon the behavior of the semi-amorphous regions.
iii. After the semi-amorphous regions are fully elongated and stretched out, the stress-
strain curve follows one more final high-stiffness (and covalent) regime; this
phenomenon occurs at strain values of around 50%. It is in this regime that the
deformation switches from semi-amorphous region influence to influence from the
beta-sheet nanocrystals. Due to the high-stiffness of the system as a whole, the beta-
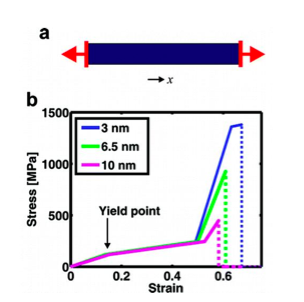
sheet nanocrystals start to sustain larger amounts of strain. As shown in figure 1b,
altering the size of the beta-sheet nanocrystals can reduce the amount of stress the
system incurs. Through experimentation performed at our lab, we can now see that
silk fibrils break at lower stress values when the size of the crystals is larger.
Consequently, the third regime lasts significantly longer when the crystal size is
smaller.
iv. Finally, failure of the spider silk occurs when the applied force reaches the
maximum tensile strength of the silk (the maximum tensile strength varies for
different systems and parameters). One visible sign of failure includes some
individual beta-strands being completely pulled out. To reinforce how strong spider
silk is, it is important to note that failure occurs on approximately the order of a GPa.
Like in the third regime, the point of failure is determined entirely by the strength of
the beta-sheet nanocrystals, which is directly proportional to their size.
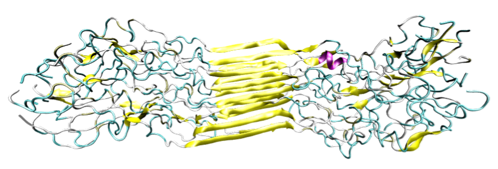
Figure 2: Molecular Nanostructure of Silk Protein Domain
This image from a replica exchange simulation displays the twisted, hierarchical structure of spider silk on a nanoscale level. The middle region makes up the beta-sheet nanocrystals, with the ancillary regions represent amorphous residues. We can see the orderly stacking formation of the beta-sheet nanocrystals in the middle region of the molecule. However, the other portion of the molecule displays the semi-amorphous regions of spider silk, with the much less organized structure (adapted from Keten et al., 2010 [2]).
Figure 3: Representation of spider silk nanostructure under increasing deformation
levels.
This picture is a representation of the spider silk unit cell’s nanostructure under varying (increasing) levels of deformation. The nanostructure consists of the depicted yellow beta-sheet nanocrystals as well as the surrounding semi-amorphous matrix. The purpose of applying increasing levels of deformation to the structure is to determine the mechanical response of spider silk to deformation all
the way until complete failure (adapted from Keten et al., 2010 [2]).
This simulation is based on values from simulations and models developed by graduate students Sinan Keten and Andrea Nova from the Laboratory of Atomistic and Molecular Mechanics.
Figure 1: Idealized representation of spider silk nanostructure under tensile loading (from A. Nova et al., 2010 [1]); (a) Schematic of model setup and loading geometry of tensile testing; (b) Comparison of beta-sheet nanocrystal size effect on the stress-strain response.