The Notation of Differentiation

A derivative is always the derivative of a function with respect to a variable. When we write the definition of the derivative as

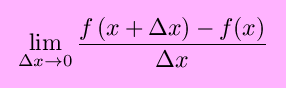
we mean the derivative of the function f(x) with respect to the variable x.

One type of notation for derivatives is sometimes called prime notation. The function f´(x), which would be read ``f-prime of x'', means the derivative of f(x) with respect to x. If we say y = f(x), then y´ (read ``y-prime'') = f´(x). This is even sometimes taken as far as to write things such as, for y = x⁴ + 3x (for example), y´ =  (x⁴ + 3x)´.
Higher order derivatives in prime notation are represented by increasing the number of primes. For example, the second derivative of y with respect to x would be written as

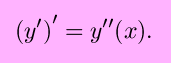
Beyond the second or third derivative, all those primes get messy, so often the order of the derivative is instead writen as a roman superscript in parenthesis, so that the ninth derivative of f(x) with respect to x is written as f⁽⁹⁾(x) or f^(ix)(x).

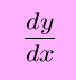
(his is read as ``dy -- dx'', but not ``dy minus dx'' or sometimes ``dy over dx''). Since y = f(x), we can also write

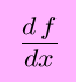
This notation suggests that perhaps derivatives can be treated like fractions, which is true in limited ways in some circumstances. (For example with the chain rule.) This is also called differential notation, where dy and dx are differentials. This notation becomes very useful when dealing with differential equations.

A variation of Leibnitz's differential notation is written instead as

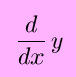
which resembles the above operator notation, with (d/dx as the operator).

Higher order derivatives using leibnitz notation can be written as

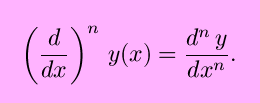
The exponents may seem to be in strange places in the second form, but it makes sense if you look at the first form.