

|
|
| Thermodynamics and Propulsion | |
Subsections
13.3 Aircraft Range: the Breguet Range Equation
Consider an aircraft in steady, level flight, with weight
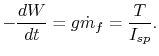
For steady, level flight,
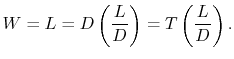
The rate of change of aircraft gross weight is thus
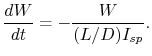
Suppose
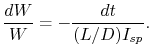
We can integrate this equation for the change in aircraft weight to yield a relation between the weight change and the time of flight:
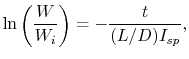
where

The range is the flight time multiplied by the flight speed, or,

The above equation is known as the Breguet range equation. It shows the influence of aircraft, propulsion system, and structural design parameters.
13.3.1 Relation of overall efficiency,
Suppose
| |||||||||
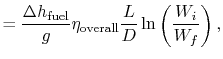 |
||
|
or
| ||
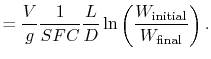 |
||
The combustion efficiency is near unity unless conditions are far off design. We can therefore regard the two main drivers as the thermal and propulsive13.1 efficiencies. The evolution of the overall efficiency of aircraft engines in terms of these quantities was shown in Figure 11.8.
UnifiedTP