Energy Integral.

Next: Approximate Method Based on Up: Momentum and Energy Equations. Previous: Momentum Integral

Energy Integral.

We multiply equation (4.77) and (4.78) respectively by 2u and (U²-u²), so we have

$\begin{displaymath}% latex2html id marker 1024 \mbox{(\ref{eq:ch234b})} \rightar... ...frac{\partial u}{\partial x}-2vu\frac{\partial u}{\partial y}, \end{displaymath}$

(94)

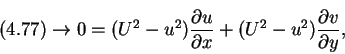$\begin{displaymath}% latex2html id marker 1039 \mbox{(\ref{eq:ch234b})} \rightar... ...ial u}{\partial x}+(U^{2}-u^{2})\frac{\partial v}{\partial y}, \end{displaymath}$

(95)

and by adding them we obtain

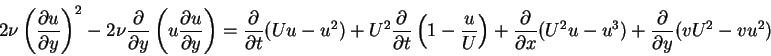$\begin{displaymath}2\nu\left(\frac{\partial u}{\partial y}\right)^{2}-2\nu\frac{... ...al x}(U^{2}u-u^{3})+\frac{\partial}{\partial y}(vU^{2}-vu^{2}) \end{displaymath}$

(96)

If we integrate the equation above with respect to y from 0 to $\infty$ , and if we take into account equations (4.79) to (4.83), we obtain

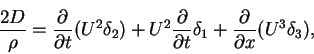$\begin{displaymath}\frac{2D}{\rho} = \frac{\partial}{\partial t}(U^{2}\delta_{2}... ...ial t}\delta_{1}+\frac{\partial}{\partial x}(U^{3}\delta_{3}), \end{displaymath}$

(97)

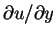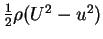since v(U²-u²) and $\partial u/\partial y$ both tend to zero as y tends to infinite and the term (vU²)_y=0 is zero because it is assumed that there is no suction on the plate. The energy integral may also be regarded as an equation for the ``kinetic energy defect'' $\frac{1}{2}\rho(U^{2}-u^{2})$ per unit volume, namely

$\begin{displaymath}\frac{\partial}{\partial t}\int_{0}^{\infty}\frac{1}{2}\rho(U... ... = D+\rho\frac{\partial U}{\partial t}\int_{0}^{\infty}(U-u)dy \end{displaymath}$

(98)

Next: Approximate Method Based on Up: Momentum and Energy Equations. Previous: Momentum Integral

Karl P Burr
2003-03-12