6) Wettability, non-wettability and contact angle hysteresis
Wetting refers to the study of how a liquid deposited on a solid (or liquid) substrate spreads out. Understanding wetting enables us to explain why water spreads readily on clean glass but no on a plastic sheet.
(i) Example: 'Total wetting' vs. 'Non-wetting'
When a drop is placed down on very clean glass, it spreads completely. By contrast, the same drop deposited on a sheet of plastic remains stuck in its place. The conclusion is that there exist two regimes of wetting. Wetting can be characterized into two types:
total wetting: when the liquid has a strong affinity for the solid; and
partial wetting, the opposite case.
|
|
|
|
| water on cleaned glass slide |
on nanotube carpet |
Click here for the videos: hydrophilic, ultrahydrophobic
(ii) Spreading Parameter
Spreading parameter, S distinguishes the two different regimes of wetting. It measures the difference between the surface energy (per unit area) of the substrate when dry and wet:
S = [Esubstrate]dry - Esubstrate]wet or S = γsolid - (γliquid + γsolid-liquid)
S > 0: Total wetting
If the parameter S is positive, the liquid spreads completely in order to lower its surface energy. Condition favorable for this condition is a high value of γsolid (high energy surfaces like glass, clean silicon) and a lower value of γliquid (ethanol, toluene). [See above figure]
S < 0: Partial wetting
The drop does not spread but, instead, forms at equilibrium a spherical cap resting on the substrate with a contact angle θ. A liquid is said to be "mostly wetting" when θ < 90°, and "mostly non-wetting" when θ > 90°.
(iii) Partially wetting and partially non-wetting
 |
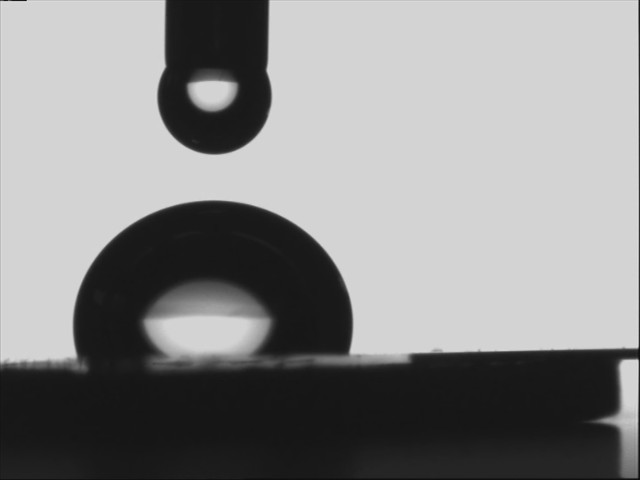 |
| Water on polymethylmethacrylate | water on silanized silicon wafer |
Click here for the videos: partially wetting, hydrophobic
When the solid has a high affinity for water - in which case it is called hydrophilic (high energy e.g. glass)- water spreads. In the opposite case of hydrophobic (low energy e.g. teflon) surfaces, water does not spread but, instead, forms at equilibrium a spherical cap resting on the substrate with a 'contact angle'.
(iv) Young's equation for contact angle
The contact angle can be obtained via one of two methods
1) The first method consists in tallying up the capillary forces acting on the line of contact (also called triple line) and equating the sum to zero. When normalized to a unit length, these forces are the interface tensions between the three phases (S/L/G). By projecting the equilibrium forces on the solid plane, one obtains the Young' relation.
γsl - γs + γlv.cos θ = 0 or cos θ = (γs - γsl )/ γlv
It is evident that θ can be defined only if the spreading parameter is negative. θ increases when the liquid is non-wetting.
2) The second method relies on calculating the work done by moving the line of contact by a distance dx:
dW = γsl.dA - γs.dA + γlv.dA.cos θ
At equilibrium, dW/dA = 0, which leads to the same equation as above. For information about measurement of contact angle, click here.
(v) Contact angle hysteresis: advancing Vs receding contact angle
Contact angle measured for a liquid advancing across a surface exceeds that of one receding from the surface. Contact angle is generally attributed to surface roughness, surface heterogeneity, solution impurities adsorbing on the surface, or swelling. Advancing contact angle (θA < θR) is always larger than or equal to the receding contact angle. The difference between the two is called contact angle hysteresis.
Advancing and Receding Contact Angles
|
|
|
|
| Advancing CA |
|
Receding CA |
Click here for the videos: Advancing contact angle, Receding contact angle
If we measure the contact angle while the volume of the drop is increasing - practically this is done just before the wetting line starts to advance-we get the so called advancing contact angle θA. If we afterwards decrease the volume of the drop and determine the contact angle just before the wetting line is receding, we measure the so called receding contact angle, θR. Usually θA is significantly higher than θR. The difference θA - θR is called contact angle hysteresis.
Drop running down a plane:
A liquid drop can remain stuck on a tilted plane, despite gravity, if the contact angle is different at the front and at the rear of the drop (contact angle hysteresis). The rear angle being smaller than the front one, a capillary force exists, which opposes the gravity force. The condition for the drop to be stuck on the solid is
πr γ (cosθR-cosθA) > ρV g sin α
r is the radius of the contact line (considered as circular), γ and ρ are the surface tension and density of the liquid, g is the gravity acceleration, V is the volume of the drop, α is the angle of the plane. For more information on drops at rest on tilted plane, click here. A commonplace example of this phenomena is rain drop falling down from car windscreens.
Water on silanized silicon surface
(vi) Practical consequences
-Non-stick frying pans are coated with teflon, a low energy surface and hence poorly wetted surface.
-rain coats are made of hydrophobic material so that rain drops don't stick to the surface and can be removed by just shaking the rain coat.
-wax paper cups from McDonalds are hydrophobic, so that your coke doesn't stick to the cup and hence, the cup appears shiny.
(vii) Drop sliding on hydrophobic surface
Hydrophobic surfaces are desired for water proof materials like raincoats, backpack etc. Hydrophobic surfaces also find application in what is growing in popularity, self-cleaning surfaces. This concept relies on the spherical droplets rolling off the surface and picking up particular dirt in their path without leaving any water droplets on the surface.
|
|
| drop sliding off the hydrophobic surface (parafilm) |
Back to index ||Introduction to surface tension ||Definition of surface tension||Interfacial tension ||Minimal surfaces ||Soap bubbles, Surfactants and Detergents
Wettability||Role of roughness ||Capillarity and gravity||Dynamical effects and instability||Special interfaces ||Current research in wettability
Back to NIRT Home||On our current research ||More on Wetting||Contact Angle Measurement||Atomic Force Microscopy ||My personal homepage